Question
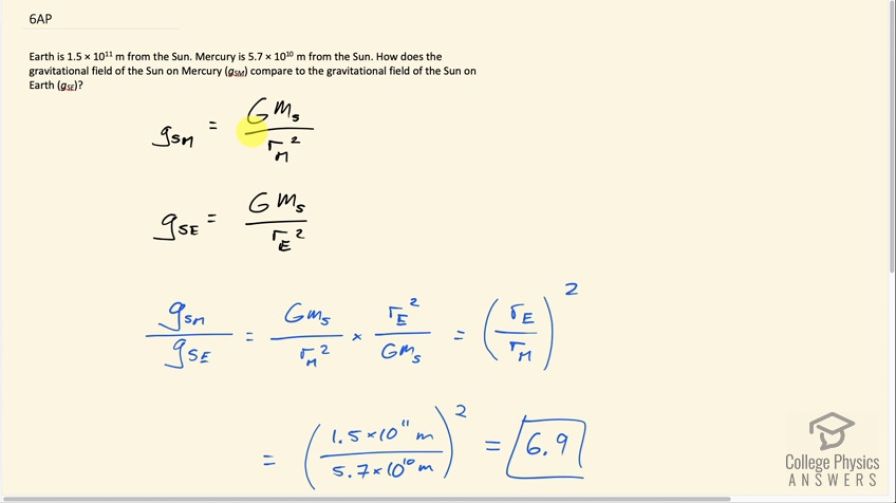
Earth is $1.5\times 10^{11}\textrm{ m}$ from the Sun. Mercury is $5.7\times 10^{ 10}\textrm{ m}$ from the Sun. How does the gravitational field of the Sun on Mercury ($g_{SM}$) compare to the gravitational field of the Sun on Earth ($g_{SE}$)?
Final Answer
The graviational field of the sun at Mercury is greater than that at Earth by a factor of 6.9.
Solution Video
OpenStax College Physics Solution, Chapter 6, Problem 6 (Test Prep for AP® Courses) (1:14)
Sign up to view this solution video!
View sample solutionCalculator Screenshots
