Question
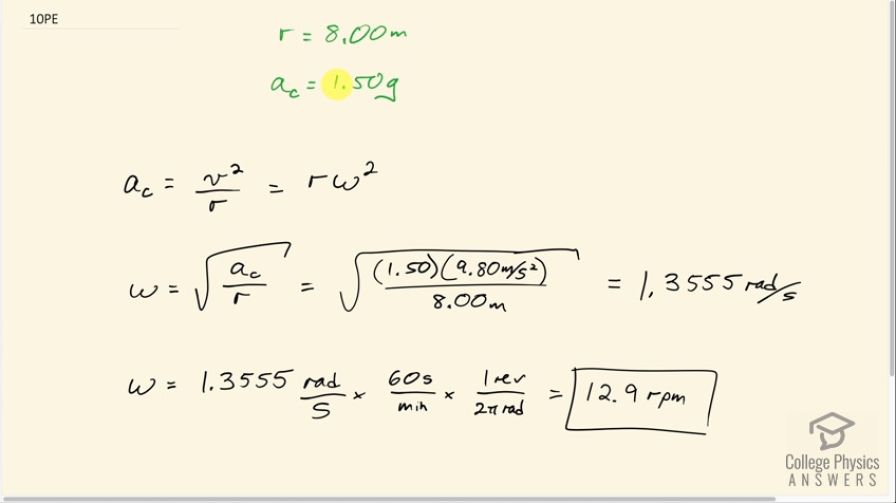
A fairground ride spins its occupants inside a flying saucer-shaped container. If the horizontal circular path the riders follow has an 8.00 m radius, at how many revolutions per minute will the riders be subjected to a centripetal acceleration whose magnitude is 1.50 times that due to gravity?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 6, Problem 10 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. This fair ground ride spins the occupants in a circle of radius 8.0 meters with an acceleration 1.50 times g. So we have to find the angular speed of this ride in rpm, or revolutions per minute. So centripetal acceleration is v squared over r but a better way to write it for this question is r times angular speed squared and then we can solve for this ω by dividing both sides by r... divide this by r and divide the acceleration by r and you are left with ω equals acceleration over r square rooted because we had to square root both sides to solve for ω. So it's gonna be the square root of 1.5 times 9.80 meters per second squared—that is the centripetal acceleration— divided by the radius of the path of the occupants which is 8.0 meters and square root that and you get 1.3555 and the units are radians per second. Then we convert that into revolutions per minute by multiplying by 60 seconds per minute. So that puts units of minutes in the denominator and then multiply by 1 revolution for every 2π radians and so we have 1.3555 times 60 divided by 2π gives us 12.9 revolutions per minute.
