Question
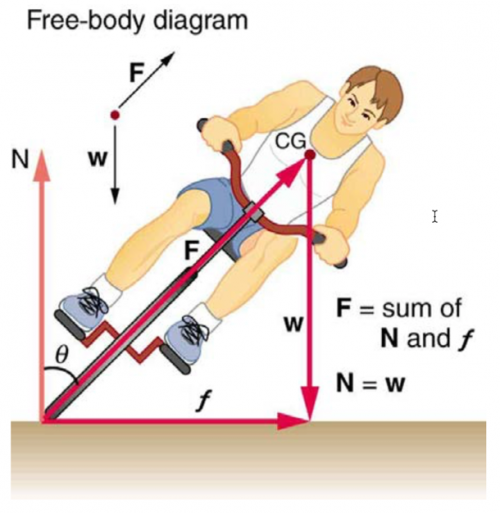
Part of riding a bicycle involves leaning at the correct angle when making a turn, as seen in Figure 6.36. To be stable, the force exerted by the ground must be on a line going through the center of gravity. The force on the bicycle wheel can be resolved into two perpendicular components—friction parallel to the road (this must supply the centripetal force), and the vertical normal force (which must equal the system's weight). (a) Show that θ\theta (as defined in the figure) is related to the speed vv and radius of curvature rr of the turn in the same way as for an ideally banked roadway—that is, θ=tan⁡−1v2rg\theta = \tan^-1{\dfrac{v^2}{rg}} (b) Calculate θ\theta for a 12.0 m/s turn of radius 30.0 m (as in a race).
A bicyclist negotiating a turn on level ground must lean at the correct angle.The net external force on the system is the centripetal force. The vertical component of the force on the wheel cancels the weight of the system while its horizontal component must supply the centripetal force. This process produces a relationship among the angle θ , the speed v , and the radius of curvature r of the turn similar to that for the ideal banking of roadways.
A bicyclist negotiating a turn on level ground must lean at the correct angle.The net external force on the system is the centripetal force. The vertical component of the force on the wheel cancels the weight of the system while its horizontal component must supply the centripetal force. This process produces a relationship among the angle θ , the speed v , and the radius of curvature r of the turn similar to that for the ideal banking of roadways.
Question by OpenStax is licensed under CC BY 4.0
Final Answer
  1. see video for derivation
  2. 26.1∘26.1^\circ

Solution video

OpenStax College Physics, Chapter 6, Problem 28 (Problems & Exercises)

OpenStax College Physics, Chapter 6, Problem 28 (PE) video thumbnail

In order to watch this solution you need to have a subscription.

Start free trial Log in
vote with a rating of votes with an average rating of .
Chevron left Prev
Select problem
Next Chevron right

Calculator Screenshots

  • OpenStax College Physics, Chapter 6, Problem 28 (PE) calculator screenshot 1
Video Transcript
This is College Physics Answers with Shaun Dychko. Our job in this question is to figure out what is this angle that the cyclist is tilted over compared to vertical. We know that there are two forces being applied at this point here. One force is straight upwards, this is a normal force and it has to be equal in magnitude to the weight of the cyclist. There's also this friction force directed this way parallel to the ground, which is providing the centripetal force that makes the cyclists go in a circle. This resultant here has components that are the normal force, and the other component is the friction force along here, and this angle then is going to be the inverse tangent of the friction force divided by the normal force. One step at a time now. First we'll say that the sum of the vertical forces then is the normal force upwards minus the weight downwards mg. That equals mass times the vertical acceleration but there's no vertical acceleration. This equals zero. We can say after we add mg to both sides, we can say the normal force equals the weight. Then considering the x-direction, we have only the friction force acting in the horizontal direction. That's going to equal mass times its horizontal acceleration, which in this context is called centripetal acceleration. That is substituted with v squared over r. We can say that the friction force then is mv squared over . We redo this triangle here and said that the tangent of this angle theta is going to be the opposite friction force divided by the adjacent, which is the normal force, and that's mv squared over r is the friction force, and then divided by the normal force. Now because this friction force is a fraction, I don't like to divide a fraction by yet another fraction because that gets confusing. So instead of multiplying by the reciprocal of the normal force, multiplying by the reciprocal of this, which is one over mg, and the m's cancel, leaving us with v squared over rg. Then if we take the inverse tangent of both sides, we solve for theta on the left and then we're left with this expression on the right. Theta is inverse tangent of v squared over radius of curvature of its circle, times g. If a cyclist was traveling at 12 meters per second, we'd have to square that and divide by the radius of the circle he's traveling in, which is 30 meters times acceleration due to gravity, 9.8 meters per second squared, take the inverse tangent of all that and we'd get this tilt would be 26.1 degrees.