Question
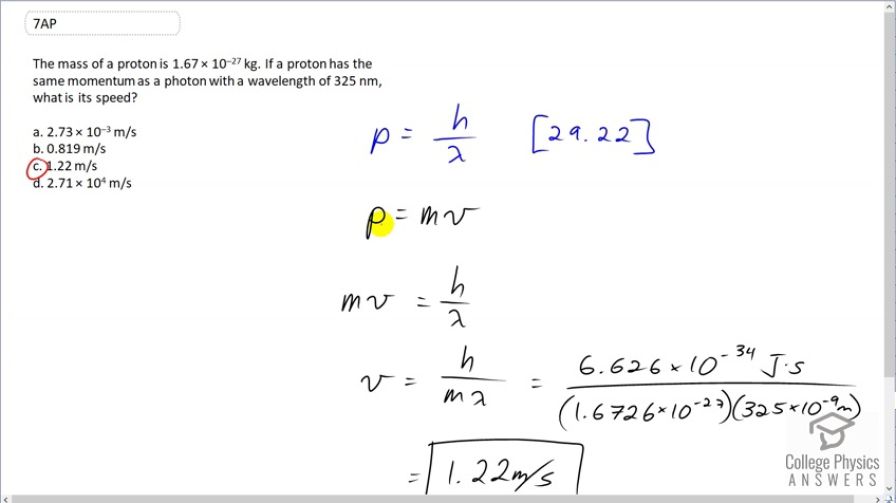
The mass of a proton is 1.67×10−27 kg1.67\times 10^{-27}\textrm{ kg}. If a proton has the same momentum as a photon with a wavelength of 325 nm, what is its speed?
  1. 2.73×10−3 m/s2.73\times 10^{-3}\textrm{ m/s}
  2. 0.819 m/s0.819\textrm{ m/s}
  3. 1.22 m/s1.22 \textrm{ m/s}
  4. 2.71×104 m/s2.71\times 10^{4}\textrm{ m/s}
Question by OpenStax is licensed under CC BY 4.0
Final Answer

(c)

Solution video

OpenStax College Physics for AP® Courses, Chapter 29, Problem 7 (Test Prep for AP® Courses)

OpenStax College Physics, Chapter 29, Problem 7 (AP) video thumbnail

In order to watch this solution you need to have a subscription.

Start free trial Log in
vote with a rating of votes with an average rating of .
Chevron left Prev
Select problem
Next Chevron right

Calculator Screenshots

  • OpenStax College Physics, Chapter 29, Problem 7 (AP) calculator screenshot 1
Video Transcript
This is College Physics Answers with Shaun Dychko. The momentum of a photon is Planck's constant divided by its wavelength— that's equation [29.22]. The momentum of a proton moving at a non-relativistic speed is its mass times its velocity. So let's equate these two things— mv and h over λ to figure out what the speed of the proton would be in order to have the same momentum as this photon of wavelength 325 nanometers. So we'll divide both sides by m here and we get that the speed then is Planck's constant over m times the wavelength. So that's Planck's constant divided by mass of the proton times the wavelength of 325 times 10 to the minus 9 meters which is the speed of 1.22 meters per second.