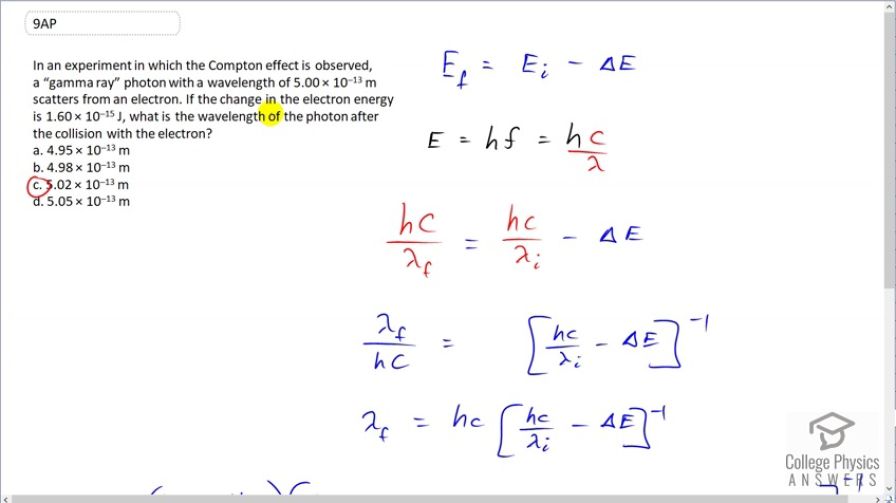
Question
In an experiment in which the Compton effect is observed, a “gamma ray” photon with a wavelength of scatters from an electron. If the change in the electron energy
is , what is the wavelength of the photon after the collision with the electron?
Final Answer
(c)
Solution video
OpenStax College Physics for AP® Courses, Chapter 29, Problem 9 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
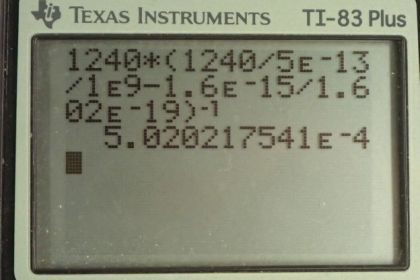
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A photon with an initial wavelength of 5.00 times 10 to the minus 13 meters hits an electron and it causes the electron to gain 1.60 times 10 to the minus 15 joules of energy and so the question is what is the wavelength of the photon after this collision? So the energy that the electron gained came at the expense of the photon's energy. So the photon's energy has been reduced by this much and so what wavelength will the photon have after having this much energy taken away? So the final energy of the photon will be its initial energy that we can calculate using hf—that's the energy of a photon— minus the energy that has been lost to the electon, we'll call it ΔE. And we can replace f with c over λ because we are given wavelengths here and we are asked for what the wavelength is so we have to express our energy in terms of wavelength. So we have hc over λ is energy of a photon and the final energy will be hc over the final wavelength that we want to find and that equals the hc over the initial wavelength that we are given minus the energy lost to the electron. So let's raise both sides to the exponent negative 1 in order to put this λ f in the numerator and on the right hand side, we'll just put a bracket around it and write the exponent negative 1 and on the left side, we flip the fraction so that's λ f over hc. And then we'll solve for λ f by multiplying both sides by hc. So the final wavelength is gonna be Planck's constant times speed of light times bracket Planck's constant speed of light over the initial wavelength minus the energy lost to the electron, all to the exponent negative 1. So that's 1240 electron volt nanometers; that's what the product of Planck's constant times c is, and that's multiplied by 1240 electron volt nanometers divided by 5.00 times 10 to the minus 13 meters—initial wavelength— but we have to write this in units of nanometers in order to cancel with the nanometers that are in our numerator here. So we multiply by 10 to the 9 nanometers per meter. And so then these nanometers cancel and then minus 1.60 times 10 to the minus 15 joules but we have to write this energy in units of electron volts, in order to match with the electron volts here so we multiply by 1 electron volt for every 1.602 times 10 to the minus 19 joules and this difference gets raised to the exponent negative 1 multiplied by 1240 and we get 5.02 times 10 to the minus 4 nanometers which we'll write in units of meters since all of our possibilities are written in meters. So we times by 1 meter for every 10 to the 9 nanometers. That's 5.02 times 10 to the minus 13 meters; we expected to get a wavelength that is longer than what we started with because this photon has lost energy to the electron and by losing energy, we expected its wavelength to get bigger and so it should be greater than 5.00 and the only options that fit that description are (c) and (d) and well (c) exactly matches what our answer is and so that is the answer.