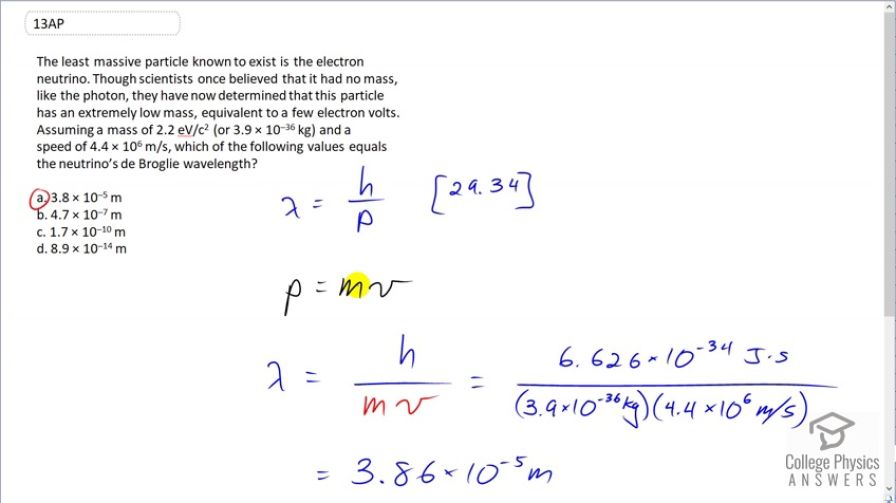
The least massive particle known to exist is the electron neutrino. Though scientists once believed that it had no mass, like the photon, they have now determined that this particle has an extremely low mass, equivalent to a few electron volts. Assuming a mass of $2.2{\text{ eV/c}}^2$ (or $3.9\times 10^{-36}\text{ kg}$) and a speed of $4.4\times 10^6\text{ m/s}$, which of the following values equals the neutrino’s de Broglie wavelength? (a) $3.8\times 10^{-5}\text{ m}$
(b) $4.7\times 10^{-7}\text{ m}$
(c) $1.7\times 10^{-10}\text{ m}$
(d) $8.9\times 10^{-14}\text{ m}$

Video Transcript

This is College Physics Answers with Shaun Dychko. The de Broglie wavelength is Planck's constant divided by momentum. So this electron neutrino will have a non-relativistic momentum since this speed is only times 10 to the 6 meters per second whereas speed of light is 3 times 10 to the 8. So we can use mv for our momentum formula and substitute that in, which we do here. And so the wavelength is Planck's constant divided by the mass of the electron neutrino times its speed. So that's Planck's constant divided by 3.9 times 10 to the minus 36 kilograms times 4.4 times 10 to the 6 meters per second which is a wavelength of 3.86 times 10 to the minus 5 meters which is answer (a).