Question
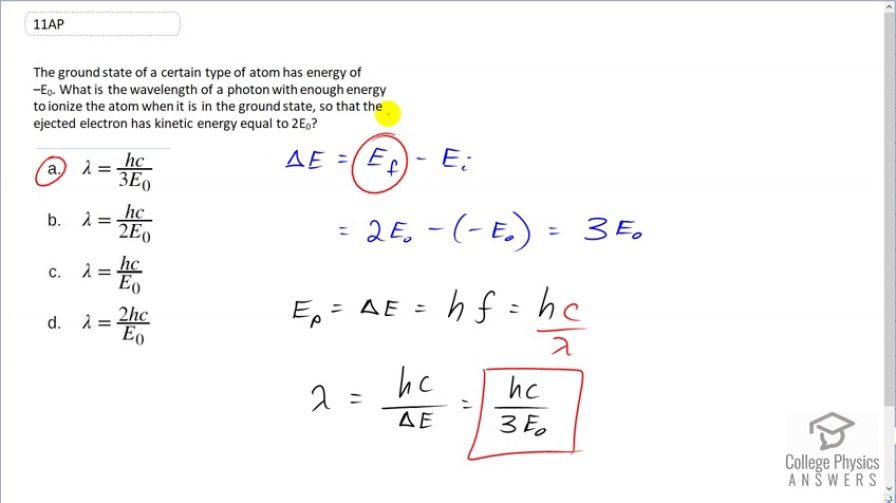
The ground state of a certain type of atom has energy of −Eo-E_o. What is the wavelength of a photon with enough energy to ionize the atom when it is in the ground state, so that the ejected electron has kinetic energy equal to 2Eo2E_o?
  1. λ=hc3Eo\lambda = \dfrac{hc}{3E_o}
  2. λ=hc2Eo\lambda = \dfrac{hc}{2E_o}
  3. λ=hcEo\lambda = \dfrac{hc}{E_o}
  4. λ=2hcEo\lambda = \dfrac{2hc}{E_o}
Question by OpenStax is licensed under CC BY 4.0
Final Answer

(a)

Solution video

OpenStax College Physics for AP® Courses, Chapter 29, Problem 11 (Test Prep for AP® Courses)

OpenStax College Physics, Chapter 29, Problem 11 (AP) video thumbnail

In order to watch this solution you need to have a subscription.

Start free trial Log in
vote with a rating of votes with an average rating of .
Chevron left Prev
Select problem
Next Chevron right
Video Transcript
This is College Physics Answers with Shaun Dychko. The change in energy of this atom is going to be the final energy minus the initial energy. And so we are told that it is ionized when it has an energy of 2 times E naught and so the final energy is 2 times E naught. And we are told that it starts in the ground state of energy negative E naught and so we substitute negative E naught in here. This works out to 3 E naught being the change in energy. So we need a photon with this energy. And the energy of a photon is hf, which we'll write in terms of wavelength by writing c over λ in place of f and we'll solve for λ by multiplying, well this by λ divided by ΔE and then multiply this by λ over ΔE. So λ is hc over ΔE and ΔE being 3 times the E naught and that is answer (a).