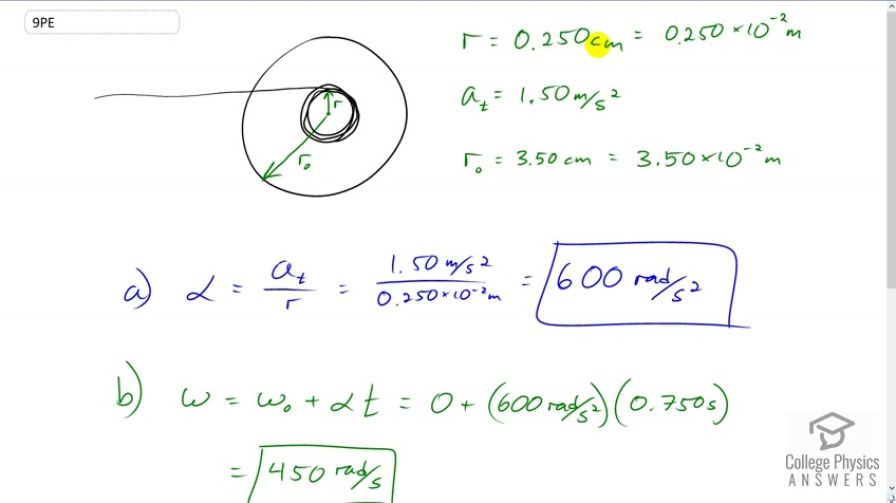
- If the string is stationary and the yo-yo accelerates away from it at a rate of , what is the angular acceleration of the yo-yo?
- What is the angular velocity after 0.750 s if it starts from rest?
- The outside radius of the yo-yo is 3.50 cm. What is the tangential acceleration of a point on its edge?
a)
b)
c)
Solution video
OpenStax College Physics, Chapter 10, Problem 9 (Problems & Exercises)
Calculator Screenshots
Comments
for part C, why do you multiply the outside radius by the angular acceleration solved for the inside radius?
Hi Jairus, thanks for the good question. Imagine a line from the center to the outside radius. It's easier to picture angular displacement, so let's talk about that. Angular displacement is the amount of angle that a radius moves as the disk rotates. Compare the angle moved by a line from the center to half-way along the radius to the angle moved by a line that is the entire radius -> they're the same! Points along a radius have the same angular displacement, and they also likewise have the same angular velocity and angular acceleration. This is why the angular acceleration for the inside radius is the same as the angular acceleration of the outside radius, so we can multiply the outside radius by the angular acceleration of the inside radius (which is the same angular acceleration as for the outside).
Hope this helps,
Shaun


