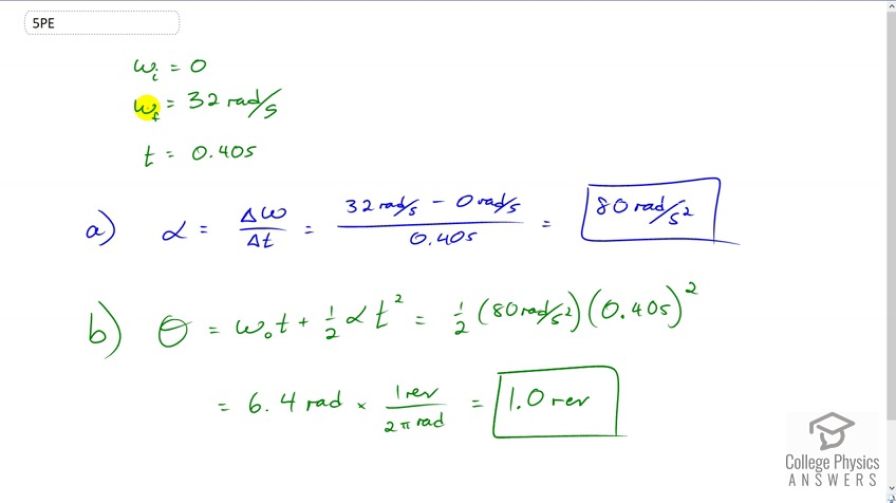
- What is its angular acceleration in rad/s2?
- How many revolutions does it go through in the process?
a)
b)
Solution video
OpenStax College Physics, Chapter 10, Problem 5 (Problems & Exercises)
Calculator Screenshots
Comments
On the calculator screenshot, I see you divided 6.4/2/pi, instead of 6.4/2pi. What's the reason for that?
Hello, thank you for the question. 6.4/2/pi is the same as 6.4/(2pi) (I think you meant to put the brackets around the denominator, which is important). The calculator evaluates left to right, so it answers 6.4/2 first, then takes that answer and divides by pi, which gives the same result as 6.4/(2pi) but with fewer button pushes on the calculator since I don't need to enter the brackets. It's just a button saving efficiency -- a personal preference in other words. Consider an example with easier numbers: 12/4/3 becomes 3 / 3 when the calculator first evaluates 12/4, and the final answer is 1. Compare this with 12/(4*3) = 12/12 = 1, just the same, but with extra button pushing. The calculator evaluates left to right with operators of equal precedence (like division/multiplication).
Hope this helps,
Shaun


