Question
Gasoline is piped underground from refineries to major users. The flow rate is (about 500 gal/min), the viscosity of gasoline is ,
and its density is . (a) What minimum diameter
must the pipe have if the Reynolds number is to be less than 2000? (b) What pressure difference must be maintained along each kilometer of the pipe to maintain this flow rate?
Final Answer
Solution video
OpenStax College Physics, Chapter 12, Problem 59 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
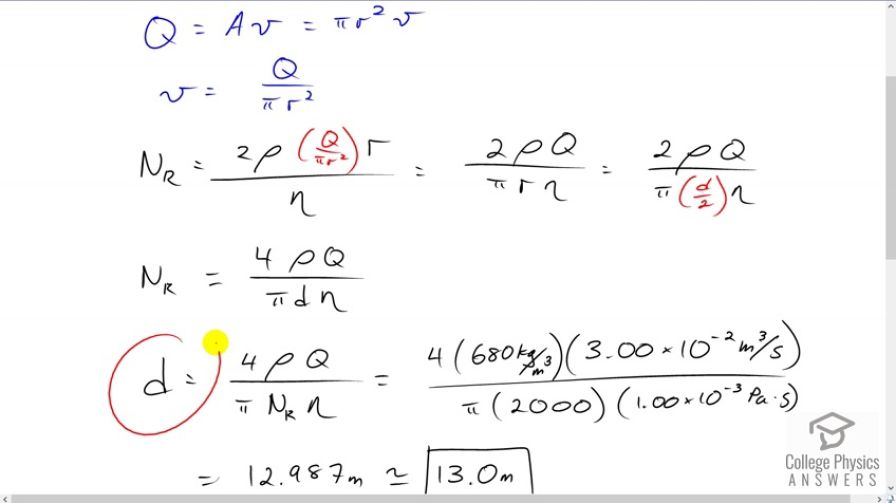
This is College Physics Answers with Shaun Dychko. Combining together the formula for Reynolds number and the formula for volume flow rate, we can solve for the diameter of the pipe and find out what is the minimum diameter such that the Reynolds number is 2000. So given the Reynolds number is two times the density of the gasoline times its speed through the pipe times the pipe’s radius divided by viscosity of gasoline, we can look at the volume flow rate equation to figure out what is v. Now the cross sectional area is pi r squared and so we can divide both sides by pi r squared to solve for v. Then we get v is the volume flow rate which we’re given, divided by pi times radius squared. So we substitute that in for v in the Reynolds number formula, and this r squared in the denominator cancels, one of them cancels with this r here, so we have r to the power of one in the denominator. We have two times density times volume flow rate divided by pi times radius of the pipe times viscosity. And then for the radius, we’ll substitute diameter divided by two. And so in this case we get Reynolds number is four times rho Q over pi times diameter times nu. Then we can solve for diameter by multiplying both sides by d over NR for Reynolds number, and we get diameter is 4 rho Q over pi NR nu. So that’s four times density of gasoline which is 680 kilograms per cubic meter times the volume flow rate of three times ten to the minus two cubic meters per second divided by pi times Reynolds number of 2000 times a viscosity of one times ten to the minus three Pascal seconds. This works out to 13.0 meters in diameter. And then part b is asking for what is the pressure difference needed along the pipe for one kilometer stretch of pipe. So this is Poiseuille's Law here that says the volume flow rate is the difference in pressure times pi times the radius of the pipe to the power of four divided by eight times the viscosity times the length of the pipe. Now we’ll solve for delta P by multiplying both sides by 8 nu l over pi r to the fourth. So we have pressure difference then is 8 nu Q l over pi r to the four. And, not sure why I repeated the formula there but nevertheless, we just plug in some numbers here. So we have eight times one times ten to the minus three Pascal seconds times three times ten to the minus two cubic meters per second, volume flow rate, times the length of one kilometer which is 1000 meters, divided by pi times the diameter we found before divided by two to get radius and raise that to the power of four, and we get 4.3 times ten to the minus six Pascal.
