Question
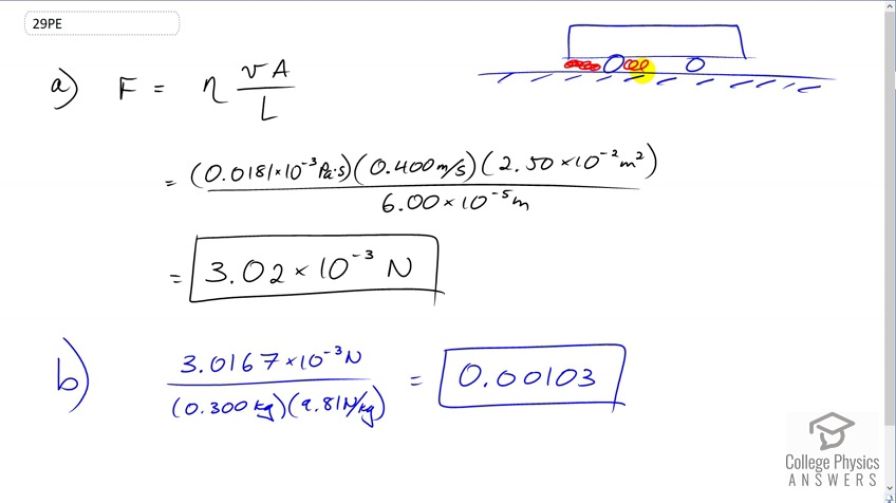
(a) Calculate the retarding force due to the viscosity of the air layer between a cart and a level air track given the following information—air temperature is 20∘C20^\circ\textrm{C}, the cart is moving at 0.400 m/s, its surface area is 2.50×10−2 m22.50 \times 10^{-2} \textrm{ m}^2, and the thickness of the air layer is 6.00×10−5 m6.00 \times 10^{-5} \textrm{ m}. (b) What is the ratio of this force to the weight of the 0.300-kg cart?
Question by OpenStax is licensed under CC BY 4.0
Final Answer
  1. 3.02×10−3 N3.02 \times 10^{-3} \textrm{ N}
  2. 0.001030.00103

Solution video

OpenStax College Physics, Chapter 12, Problem 29 (Problems & Exercises)

OpenStax College Physics, Chapter 12, Problem 29 (PE) video thumbnail

In order to watch this solution you need to have a subscription.

Start free trial Log in
vote with a rating of votes with an average rating of .
Chevron left Prev
Select problem
Next Chevron right

Calculator Screenshots

  • OpenStax College Physics, Chapter 12, Problem 29 (PE) calculator screenshot 1
Video Transcript
This is College Physics Answers with Shaun Dychko. In part a of this question, we are calculating the retarding force due to the air layer that's between the cart and the surface that it's driving on. And we find that by multiplying by the viscosity of the air, by the velocity of the cart and by the area of the bottom of the cart and then divide by the thickness of this air layer which is capital L. So we have 0.0181 times ten to the minus three Pascal seconds, that's the viscosity of air at 20 degree Celsius times by 0.4 meters per second times two and a half times ten to the minus two squared meters divided by a very thin air layer which is six times ten to the minus five meters. This gives 3.02 times ten to the minus three newtons. Then we take that value for the retarding force due to the air layer under the card and divide by the weight of the cart which is the mass times gravitational field strength, 0.3 kilograms times 9.81 newtons per kilogram, we got a ratio of 0.00103.

Comments

Hi Shaun,
In my text book I have the value of viscosity as .0181. Is this correct?

I second that comment above mine^ my textbook is also giving my viscosity value of air @ 20C as 0.0181
it does not mention anything about 10^3 (the tables usually include that info in the top if there is a sig fig thing)

Hello @phillipmoreno and @beepboop2222, thank you for the question. The textbook is a bit sneaky with how they labeled the header on this particular table. The units are labeled mPs⋅s\textrm{mPs}\cdot\textrm{s}, which means millipascal seconds. The prefix milli means ×10−3\times 10^{-3}.
Hope this helps,
Shaun