Question
Assuming that blood is an ideal fluid, calculate the critical flow rate at which turbulence is a certainty in the aorta. Take the diameter of the aorta to be 2.50 cm. (Turbulence will actually occur at lower average flow rates, because blood is not an ideal fluid. Furthermore, since blood flow pulses, turbulence may occur during only the high-velocity part of each heartbeat.)
Final Answer
Solution video
OpenStax College Physics, Chapter 12, Problem 60 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
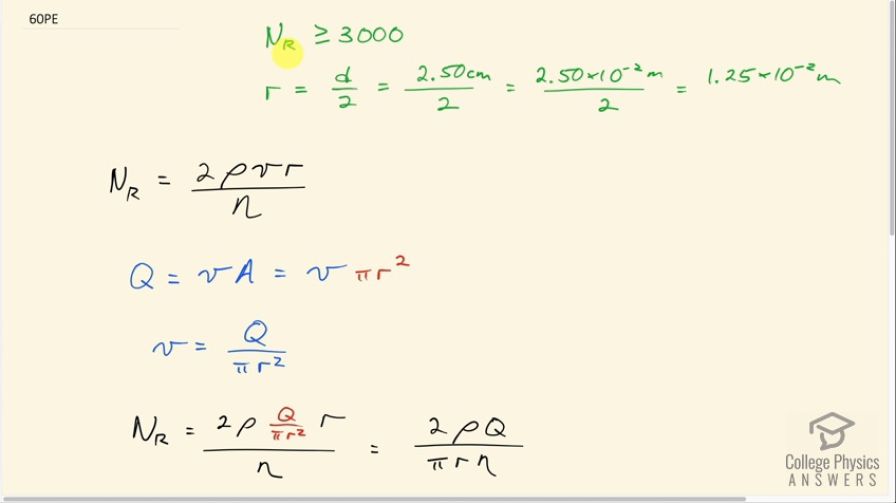
This is College Physics Answers with Shaun Dychko. We're going to calculate what volume flow rate will guarantee turbulent flow in the aorta of blood. So to guarantee the turbulent flow, you need the Reynold’s number that's more than or equal to 3000 and we're given the diameter of the aorta and we want the radius in our Reynold’s number formula. So we'll divide the diameter by two. That's two and a half centimeters divided by two, which is two and a half times 10 to the minus two meters over two, which is 1.25 times 10 to the minus two meters. So we have this Reynold’s number formula which says we multiply two by the density of blood times its speed of flow times the radius of the aorta divided by the viscosity of blood at body temperature, which is 37 degrees Celsius. Now, we're not interested in v directly. What we want to know is Q, the volume flow rate. And so that's the speed of the fluid multiplied by the cross-sectional area of the aorta, which we take to be circular. So that's gonna be pi r squared in place of the area. We can solve this for v by dividing both sides by pi r squared. And we get then that v is the volume flow rate divided by pi r squared. So we substitute that in for v in our formula for Reynold’s number. And that's what's shown in red here. And now we have an expression containing Q which we're then going to solve for. And so this r cancels with one of the r squared here, leaving us with the power of one. And then we multiply the top of this fraction by pi r and then the bottom of the fraction by pi r. This pi r cancels with this pi r and we're left with two rho Q over pi n new. So we multiply both sides by pi times radius times viscosity divided by two times density. And then that solves for Q. So, Q is pi times one and a quarter times 10 to the minus two meters radius times the viscosity of blood at 37 degrees Celsius. And so we look that up in Table 12.1, it's 2.084 Milla Pascal seconds and then the milla means multiply by 10 to the minus three. And we also need to know the density of blood, which is 1.05 times 10 to the three kilogram meters cubed. And so that's what we plugged in here for density and here for viscosity. We're multiplying by the minimum necessary Reynold's number, which is 3000. And we're end up with 1.17 times 10 to the minus four cubic meters per second is the minimum volume flow rate. That will definitely have turbulent flow.
