Question
During a marathon race, a runner's blood flow increases to 10.0 times her resting rate. Her blood's viscosity has dropped to 95.0% of its normal value, and the blood pressure difference across the circulatory system has increased by 50.0%. By what factor has the average radii of her blood vessels increased?
Final Answer
Solution video
OpenStax College Physics, Chapter 12, Problem 45 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
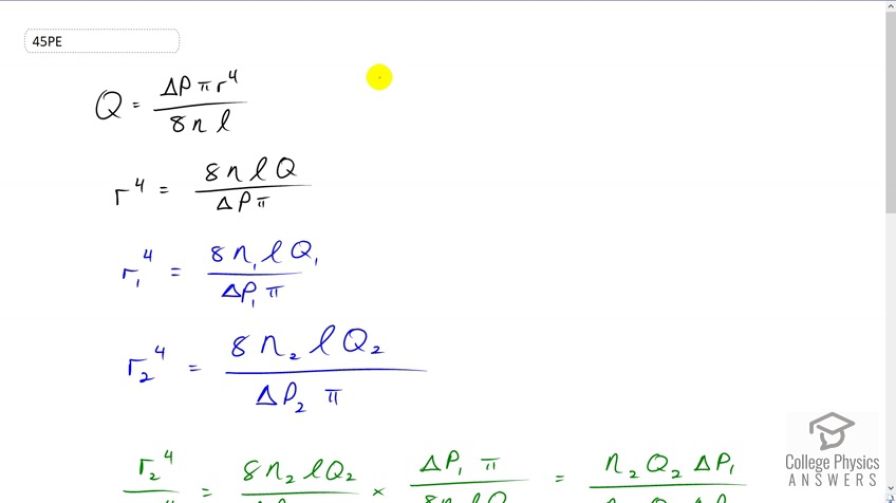
This is College Physics Answer with Shaun Dychko. When this marathoner is running,the radii of her blood vessels on average increases. We're going to figure that out by first rearranging this flow rate formula which says that there is some pressure difference that's pushing the fluid along times pi, times radius of the blood vessel to the power of four, divided by eight times the viscosity of blood, times the length of the blood vessels. That's the flow rate through the blood vessels. We'll solve this for r by multiplying both sides by eight nu l over delta p pi, and we get r to the fourth is eight nu l Q over delta p pi. So, in the first case we have all these factors the same but some of them have subscripts one on them for the first case. So we have the first viscosity, the first flow rate and the first pressure. l does not have a subscript because l is going to remain the same as she has the same length of blood vessels before and after running -- before and during the marathon. Then in the second case, of course the length of blood vessels stay the same, she doesn't grow at all but the viscosity of her blood changes with her changing body temperature and the flow rate changes and the pressure changes as well. So we divide r two to the power of four, divide it by r one to the power of four. So that's going to be this expression multiplied by the reciprocal of this expression because that's an easier way of dealing with dividing by fractions, is multiplying by its reciprocal instead. We see that a bunch of common factors cancel and we end up with the ratio of the fourth power of radii is going to be nu two Q two delta p one over nu one Q one delta p two. So we're told a few things about how things change during the marathon. So during the marathon the viscosity is 0.95 what it was before. The flow rate during the marathon is ten times what it was before. The blood pressure increases by 50 percent. So that means it is the old pressure plus 50 percent of the old pressure which works out to 1.5 times the old pressure. So now we make substitutions into this expression here. Substituting in red here we have the new viscosity, we have the new flow rate and we have the new pressure. A whole bunch of things cancel here, and we get that r two to the four over r one to the four is 0.95 times ten to the 1.5. Then we take the fourth root of both sides to get the ratio of the radii. This works out to 1.586 is the factor by which the radius changes during the marathon. So this is a 58.6 percent increase in radius.
