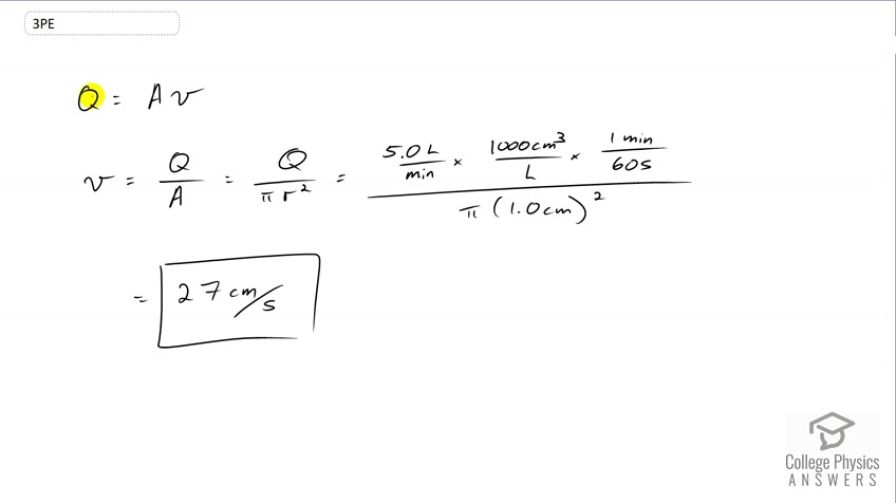
Blood is pumped from the heart at a rate of 5.0 L/min into the aorta (of radius 1.0 cm). Determine the speed of blood through the aorta.

Video Transcript

This is College Physics Answers with Shaun Dychko. We’re given the volume flow rate of blood in the aorta and we want to find its speed, also given its radius. And so we know flow rate is the cross sectional area of the blood vessel multiply by the speed of the fluid, and we’ll divide both sides by A to solve for v. And so v is Q over A. And the area is going to be pi times the radius squared. And so we have the flow rate of five liters per minute, which we convert into cubic centimeters by multiplying by 1000 cubic centimeters for every liter, and then multiply by one minute for every 60 seconds in order to end up with cubic centimeters per second here, and then we divide by pi times 1.0 centimeters squared. Now normally I would convert units into mks units, so meters, kilograms, and seconds. So we normally have meters in here, but since we have cubic centimeters in the numerator and a centimeter type unit in the denominator, the centimeter mix with the centimeter, it’s okay, there’s no need to convert them both into meters, although you could have but that’s just more work. And what ends up happening though, is we end up with an answer in centimeters per second which is kind of a nice unit here because we have a nice number of 27 centimeters per second is the speed of blood in the aorta.