Consider a parallel plate capacitor with metal plates, each of square shape of 1.00 m on a side, separated by 1.00 mm. What is the energy of this capacitor with $3.00\times 10^3\text{ V}$ applied to it? (a) $3.98\times 10^{-2}\text{ J}$
(b) $5.08\times 10^{14}\text{ J}$
(c) $1.33\times 10^{-5}\text{ J}$
(d) $1.69\times 10^{11}\text{ J}$

Video Transcript

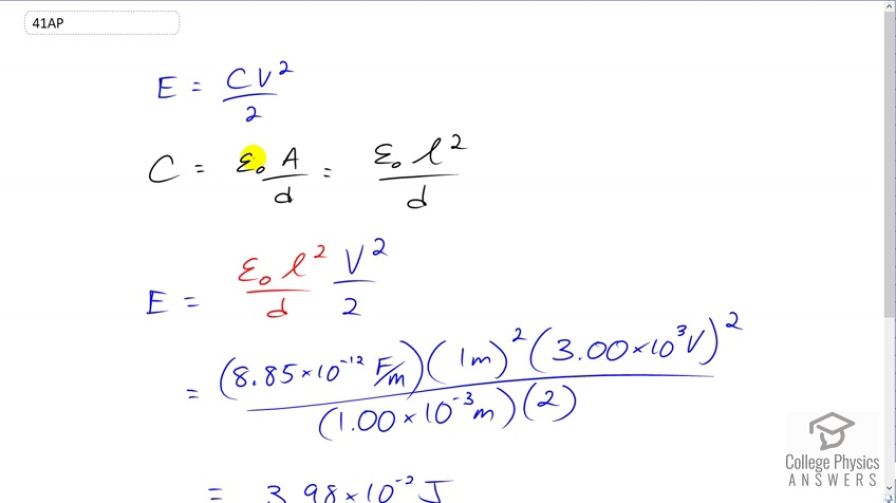
This is College Physics Answers with Shaun Dychko. The energy stored in this capacitor will be its capacitance times the voltage squared divided by two. And the capacitance for a parallel capacitors is the permittivity of free space times the area of the plates divided by the separation between them. And since it's a square plate capacitor, the area would be the side length squared. And so, we can replace C in our expression for E with this Epsilon naught L squared over D, which is what we've done here. And then, we can substitute in numbers. So, we have permittivity of free space times the side length squared, one meter squared, times 3000 volts squared, divided by the separation between the plates of one millimeter. And then, we multiply that by two, because that's what the formula says, and then we're left with 3.98 times ten to the minus two Joules, which is option A.