Question
Your friends show you an image through a microscope. They tell you that the microscope has an objective with a 0.500 cm focal length and an eyepiece with a 5.00 cm focal length. The resulting overall magnification is 250,000. Are these viable values for a microscope?
Final Answer
The image distance from the eyepiece would need to be about 100 km, which isn't realistic since a microscope can't produce the light intensity necessary to make such a distant image viewable.
Here is the Google spreadsheet used in the video.
Solution video
OpenStax College Physics, Chapter 26, Problem 32 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Video Transcript
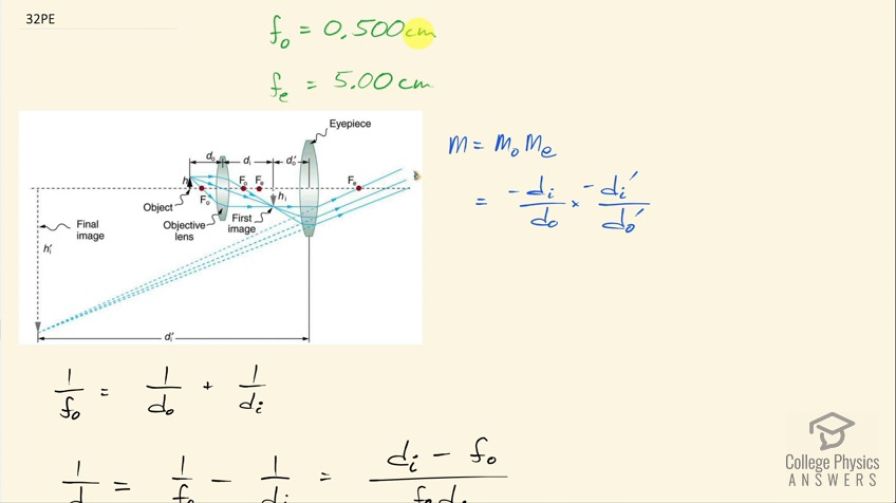
This is College Physics Answers with Shaun Dychko. We are told that a compound microscope has an objective focal length of 0.500 centimeters and an eyepiece focal length of 5.00 centimeters and an overall magnification of 250000 (so the overall magnification is 250000) and the question is are these numbers possible or viable? So the overall magnification is the product of the objective magnification and the eyepiece magnification and we are gonna figure out whether these numbers are reasonable by figuring out these image distances. This image distance here will give us a sense of how far the eyepiece and the objective are apart; the total distance between the eyepiece and the objective is d i plus this d o prime but we are just gonna solve for d i and then d i prime and get a... see if we can figure out whether these numbers are reasonable based on the values we get for d i and d i prime. Okay! So we have substituted for the magnification of the objective with negative d i divided by d o and then the magnification of the eyepiece is negative d i prime divided by d o prime and these letters are all labeled on this figure here so d o is the distance between the objective and the physical object being viewed, d i is this image distance here, d o prime is between the eyepiece and the image produced by the objective and then d i prime is the distance between the eyepiece and the final virtual image that is being viewed by the eye. So let's replace d o and d o prime... well, replace 1 over d o actually because this d i is being multiplied by 1 over d o you could say you could rewrite this as negative d i times 1 over d o and then times that by negative d i prime times 1 over d o prime so we are going to figure out what is 1 over d o and what is 1 over d o prime? First consider the objective 1 over the focal length of the objective is 1 over the object distance plus 1 over the image distance and we can solve for 1 over d o by subtracting 1 over d i from both sides. So we have 1 over d o is 1 over f o minus 1 over d i and we can write this difference as a single fraction by getting a common denominator so we multiply this fraction by the number one but of course, we are not gonna write the number one we are gonna write d i over d i and this fraction will be multiplied by f o over f o and we have d i minus f o over f o times d i is 1 over d o and then the same work can be used to figure out 1 over d o prime, it's gonna be image distance prime minus the focal length of the eyepiece divided by f e times d i prime. So this can be written in place of 1 over d o and we do that here and this can be written in place of 1 over d o prime which we do here and all of this is 250000 we are told and this d i prime cancels... or d i I should say and then this d i prime cancels and we are left with d i minus f o times d i prime minus f e all over the product of the focal lengths equals 250000. So we'll multiply both sides by f of e and then multiply these binomials together as well and so we have d id i prime minus d if e minus d i primef o plus f o times f e equals 250000 times f o times f e and then we can substitute numbers for these focal lengths and we have d id i prime minus 5 times d i minus 0.5 times d i prime equals 624997.5 square centimeters because this is 5.00 centimeters times 0.500 centimeters for each of these focal lengths I have also subtracted 5.00 times 0.500 from both sides here by the way. Okay! And then we have this line here and then factor out the d i from this term and this term. So we have d i times d i prime minus 5 equals this number plus the 0.5 times d i prime and then divide both sides by d i prime minus 5 and we have d i equals all of this in terms of d i prime and this is useful because we can make a graph or... well, actually I didn't make a graph, I just made a data table to figure out what is d i in terms of d i prime. Now there are some things that we know about these values: we know that d i is the distance between the objective and this real image and because it's a real image, this image distance must be positive or expressed with different words we could say since this image is on the opposite side of this objective lens compared to the side the object is on— that's another reason why you can say this image distance is positive. And so this d i prime well we also know something about it: we know that it's a virtual image because this image of the eyepiece is on the same side of the eyepiece as the object is for the eyepiece and so this d i prime must be negative and so we can plug in some numbers here for d i putting this formula into a Google spreadsheet and we have d i prime— we know is negative because it's the distance to a virtual image— and then we can keep plugging in different numbers until we finally get a number that is plausible for the image distance from the objective which we know has to be positive and it doesn't become positive until we plug in a massive number here which is 10 million centimeters. So somewhere between 1 million and 10 million negative centimeters, we finally get a transition from an unrealistic or impossible negative value for this image distance to a positive value. So... you know, this 10 million centimeters is 100 kilometers and so we need the image distance from the eyepiece to be about 100 kilometers before d i becomes something that's possible or in other words, positive with a value of merely 4 millimeters, which is very small and it isn't possible to see an image that's 100 kilometers away from the microscope because you have to have a very intense light production for an image that far away to be viewable and so these numbers are not realistic.