Question
In a LASIK vision correction, the power of a patient’s eye is increased by 3.00 D. Assuming this produces normal close vision, what was the patient’s near point before the procedure?
Final Answer
1.0 m
Solution video
OpenStax College Physics, Chapter 26, Problem 9 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
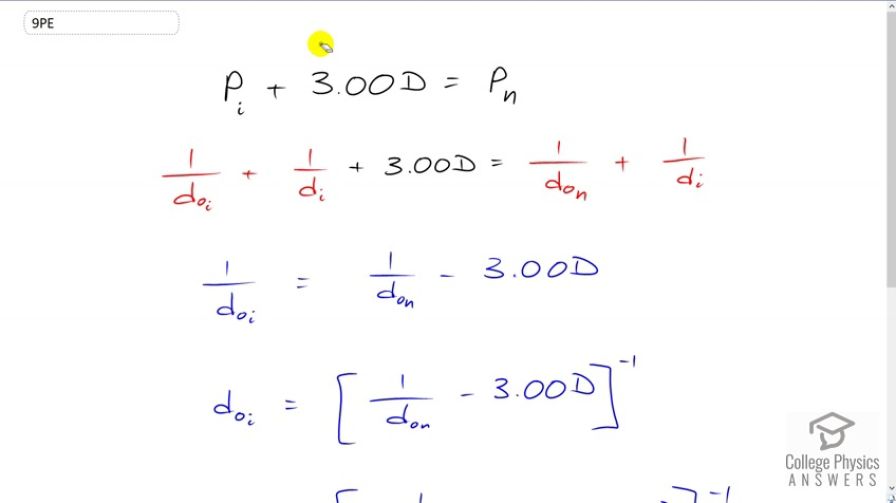
This is College Physics Answers with Shaun Dychko. This LASIK patient's eye initially had some power that was then increased by three diopters to create normal power. So, I have the subscript N for normal and a subscript I for initial. And, we want to know what is the initial near point of this person. So, we'll substitute this formula in place of the power. So, we have one over the initial near point for object distance plus one over the image distance, which is the distance between the lens and the retina. And, that's being substituted for the initial power. And then, we add three diopters to that, and it equals one over the normal object distance, which we know is 25 centimeters plus one over the image distance, which again is the lens retina distance. It doesn't change. So, this does not get a subscript initial or normal because it's the same value in each case. The lens retina distance does not change and so, this can be subtracted from both sides and so that disappears. And then, we solve for one over initial object distance by subtracting three diopters from both sides. And, we end up with one over initial object distance is one over the normal object distance minus three diopters. Then, we raise both sides to the exponent negative one, and we take the reciprocal of the left to solve for the initial object distance and that equals one over the normal object distance minus three diopters all to the power of negative one. So, that's one over 25 centimeters written as times ten to the minus two meters minus three diopters to the negative one is 1.0 meters. And, so this is their initial near point before the LASIK surgery.
