Their separation was decreased by a factor of .
Solution video
OpenStax College Physics, Chapter 18, Problem 13 (Problems & Exercises)
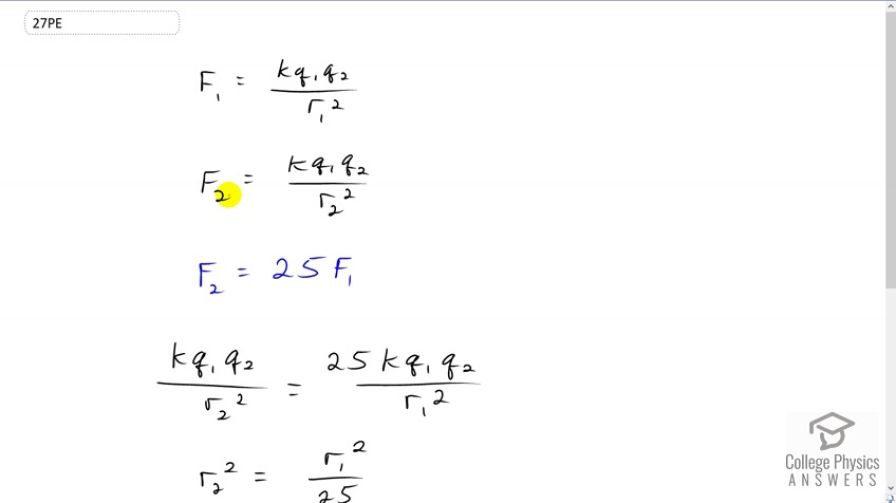
Comments
In my homework system, the correct answer was actually 5/1 or 5. I'm not sure why the discrepancy.
I think you're right Nikki although the wording gets tricky and it's hard to know exactly what they're asking or how to answer-r2 is 1/5 of r1 or in other words it takes 5 r2's to equal 1 r1. That would mean it's decreases to 1/5 it's original value but that's not the same is as being decreased by a factor of 1/5. To put actual numbers on it if r1 was 5, r2 would be 1. But the way this is answered if r1 was 5, r2 would be decreased by 1/5 that amount (1/5 of 5 is 1)and that means r2 would be (5-1) 4 and that's not right.
Hi Elizabeth, thanks again for your comment. This issue here hinges on the meaning of "decreases by a factor of..." I take the word factor to mean a number that multiplies by something else. From this point of view a factor that reduces something must be less than one, so the factor should be , not . Alternatively, it's definitely possible to see usages of the phrase "decreases by a factor of 5" with the intended meaning to divide by 5 on account of the word decreases... it really depends on the author. Definitely confusing!
All the best,
Shaun


