Solution video
OpenStax College Physics, Chapter 16, Problem 45 (Problems & Exercises)
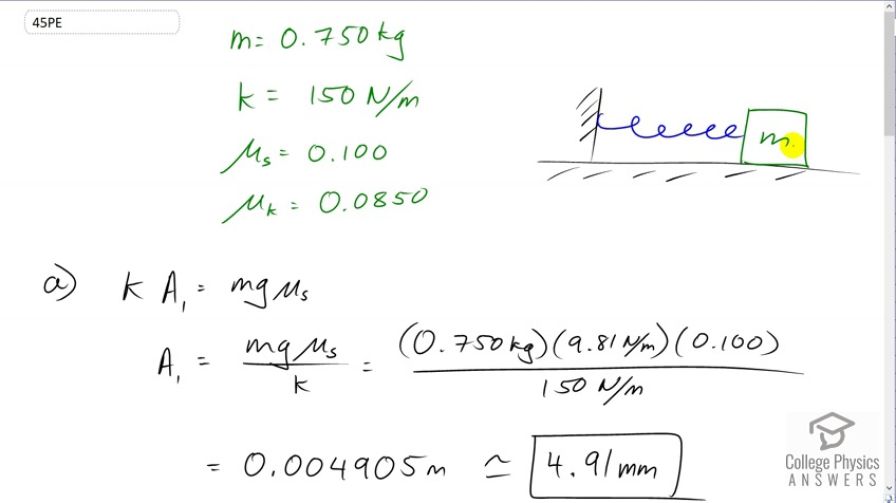
Calculator Screenshots
Comments
Hello,
When system is not moving in final position , should be energy equal to zero??.a.ka equation is 1/2k(A)^2 = Mmgd.
I think potenial energy is zero in final position because A=0-distance from equalibrium point. Where i get wrong???
Thanks.
Below is copy/pasted from an emailed question from a student that I'm including here in case it helps others:
Hi Shaun,
Problem 45 is a rather devious one in which we find the maximum distance a block can be displaced without slipping on a surface with static friction. In part (b), we displace it to twice the distance and the problem asks for the total distance the block travels before coming to a halt. I found an answer different from your own, then tried follow your work from your transcript. I wasn't able to follow your work in its entirety, but I believe I found a mistake in your reasoning. You say that the maximum amplitude occurs when the kinetic friction force is equal to the spring force. Here are two excerpts from the transcript:
"Since it's going to be moving the kinetic friction is what's going to be dampening the oscillation and it's going to be dissipating some of the energy up until the point where the spring force equals the kinetic friction force."
"It's going to be at a point where it's going to stop when the spring force which is the spring constant multiplied by the final amplitude, equals the kinetic friction force."
If the spring and friction forces were opposite one another, the position where they are equal would only demarcate where the block is not accelerating, not where its velocity is zero. Worse, if the block has overshot the equilibrium position (practically guaranteed from the problem statement), the spring and friction forces will act in the same direction, opposite the motion of the block.
One rather clear indication that something is wrong is that if we allow the coefficient of kinetic friction to approach zero in your solution, the distance traveled approaches infinity, which doesn't agree with a half-oscillation solution and fails to conserve energy. Instead, we would expect the maximum amplitude to be the same as its initial amplitude, 2*mu_s*m*g/k. It is possible that your solution considers an arbitrary number of back-and-forth oscillations, which might manifest as some kind of geometric series, but from what I've skimmed, I don't see that reasoning in there. The coefficient of static friction that was linear in part (a) turns quadratic in part (b) and it's difficult for me to reconcile the discrepancy.
My own solution is X = 2*m*g/k * ( 2*mu_s - mu_k) = 6.4 mm. This was obtained from conservation of energy principles, demanding that the initial potential energy of the spring plus the (negative) external work performed by friction be equal to the final potential energy of the spring. In equation form:
1/2*k*x_0^2 - mu_k*m*g*(x_0+x_1) = 1/2*k*x_1^2
x_0 = 2*mu_s*m*g/k
where x_0 is the initial displacement of the spring and x_1 is the final displacement, assumed to be past the equilibrium position. This turned into a rather messy application of the quadratic formula and the simplicity of my solution led me to believe either there's an easier way to approach this or I'd made a mistake along the way. It's necessary to confirm that the x_1 overshoot is less than the maximum static displacement found in part (a); otherwise, the oscillations will continue until the static friction is enough to overcome the spring force to bring the block to rest.
The fact that as mu_k approaches zero, we retrieve x_1 = x_0 gives me reason to trust my own answer. On the other hand, I'm still struggling to find an easier approach to the problem that avoids direct application of the quadratic formula, plus I'm confused about the physical interpretation of x_1 approaching zero as mu_k approaches mu_s. It may be a "coincidence".
I've included a screenshot of my own work below for your inspection. Any thoughts you can provide would be welcome. Thank you!
Hello, and thank you for this thorough analysis of the problem. Firstly, thank you for showing the shortcoming of my explanation: you're quite right that "If the spring and friction forces were opposite one another, the position where they are equal would only demarcate where the block is not accelerating, not where its velocity is zero." I should have explained that while the spring and friction forces will be equal in magnitude many times during oscillation, it's the final resting position that is of concern here. The final resting position will not be at , but instead will be at a position where the kinetic friction force equals the restoring force of the spring and there is the special case that the velocity is zero. Velocity is zero twice per oscillation when the mass is at the furthest position from equilibrium. This infinitesimally small moment in time is small enough that it's still kinetic friction in play, not static. Usually the restoring force of the spring will be greater than kinetic friction at this point and the mass will change direction and continue oscillating, but, at long last, there will come a point since the amplitude is gradually decreasing as total mechanical energy of the system is dissipated where the restoring force just equals the force of kinetic friction in magnitude (but opposite direction in this position), and the mass will no longer move. The elastic potential energy of the mass at this final position is the difference between the initial elastic potential energy and the total energy dissipated by kinetic friction during all of the oscillations. I make this point at 4:03 in the video. The total energy dissipated by kinetic friction is proportional to the total distance traveled and we can solve for that distance.
You almost wrote the accounting of energy in:
1/2*k*x_0^2 - mu_k*m*g*(x_0+x_1) = 1/2*k*x_1^2
but the error is in (x_0 + x_1) in the second term. That factor should be the total distance traveled instead of the sum of the final and initial amplitudes.
The textbook actually derives a formula for us in Example 16.7, and it can be used to verify that the solution for part (b) is correct.
Thank you again for your comments, and all the best,
Shaun