Question
An circuit with a 5.00-pF capacitor oscillates in such a manner as to radiate at a wavelength of 3.30 m. (a) What is the resonant frequency? (b) What inductance is in series with the capacitor?
Final Answer
Solution video
OpenStax College Physics, Chapter 24, Problem 39 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
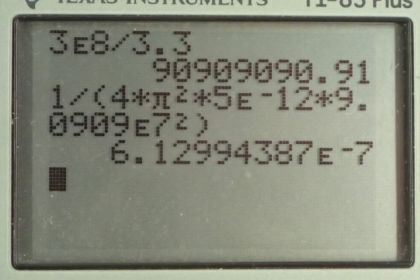
Calculator Screenshots
Video Transcript
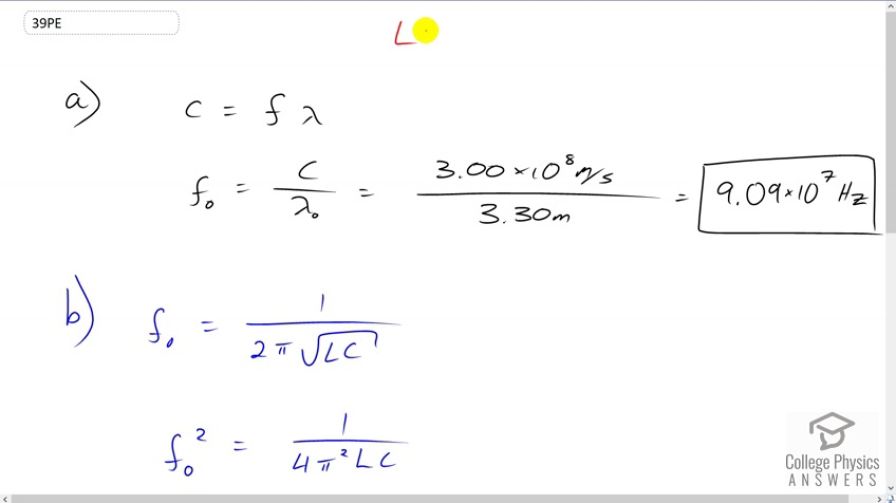
This is College Physics Answers with Shaun Dychko. We are given the wavelength of electromagnetic radiation emitted by this LR circuit or LC circuit I should say, has a Inductor in series with a capacitor. And so we have this wave equation which says that the speed of the wave which is speed of light in this case equals the frequency times wavelength and we can solve for f by dividing both sides by lambda. Now, solving for resonant frequency, so I put a subscript ‘o’ here for f naught equals the speed of light divided by the resonant wavelength, so that’s three times ten to the eight meter per second divided by 3.30 meter which is 9.09 times ten to the seven hertz. Now, the resonant frequency for an LC circuit is one over two pi times square root of the inductance times the capacitance and we can solve this for L by squaring both sides in order to remove the square root sign in denominator there and so we have f naught squared equals one over four pi squared L C and then multiply both sides by L over resonant frequency squared and we solve for the inductance here. So, L is one over four pi squared times capacitance times resonant frequency squared and so we have one our four pi squared times five times ten to the minus 12 farads because we are told that the capacitance is five pico-farads and then we multiply that by the resonant frequency we found in part A and we square that and we get 6.13 times ten to the minus seven henry is the inductance.