Question
A 200-turn flat coil of wire 30.0 cm in diameter acts as an antenna for FM radio at a frequency of 100 MHz. The magnetic field of the incoming electromagnetic wave is perpendicular to the coil and has a maximum strength of . (a) What power is incident on the coil? (b) What average emf is induced in the coil over one-fourth of a cycle? (c) If the radio receiver has an inductance of , what capacitance must it have to resonate at 100 MHz?
Final Answer
Solution video
OpenStax College Physics, Chapter 24, Problem 45 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
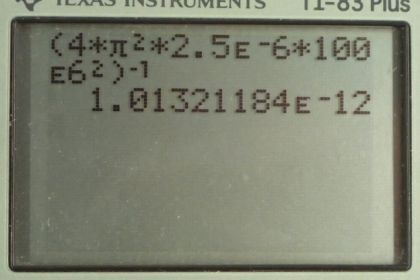
This is College Physics Answers with Shaun Dychko. A Magnetic field is perpendicular to coil and there are 200 turns in the coil we’re told and the diameter of the coil is 30 centimeters and the frequency of the electromagnetic wave, going through it, is 100 megahertz and the peak magnetic field strength is one times ten to the minus 12 Tesla. So question A , asks us for the power that is incident on the coil and that's going to be the intensity of the electromagnetic wave multiplied by the coil area. So the area is going to be Pie times r squared since it's a circular coil and we're given diameter. So we substitute diameter divided by two in place of radius and this makes Pie d Squared over four is the area. The intensity we can calculate based on knowing the peak magnetic field strength. And so it's going to be speed of light times Peak magnetic field strength squared over two times permeability of free space and we substitute this and this in place of I and A in our power formula here and then plug in numbers. So we have three times ten to the eight meters per second speed of light times one times ten to the minus 12 Tesla magnetic field strength squared times Pie times 30 times ten to the minus two meters square diameter divided by two times four Pie times ten to the minus seven Tesla meters per amp times four and we get 8.44 times ten to the minus 12 watts is the power. And the next question asks, what is the EMF that will be induced in the coil by this changing magnetic field that's coming from this electromagnetic wave. So EMF induced is change in flux divided by time. And the rate of the change of flux will be the number of turns multiplied by the area of the coil and these things don't change. So they're common factors between the two flux terms that we can factor out and then we'll have a final magnetic field minus initial magnetic field and these are the fields. This is the change in field between one quarter of a period. Now magnetic field for sinusoidally varying field is going to be the peak multiplied by sine of the angular frequency Omega times time and we need to figure out what to substitute into your now. So for the period it's going to be the reciprocal of frequency. And that's going to be useful in our final time that we substitute in here because we want to find out what is a final magnetic fields and then minus initial magnetic fields. So right now we're figuring out how to calculate Bf and so we need tf to do that because Bf is going to equal B naught times sine Omega tf and it's going to be one quarter period because the question says that what average EMF is induced over one fourth of a cycle. And then for period we can substitute in this reciprocal frequency. So this works out to one over four times frequency is the final time. Angular frequency is two Pie times frequency. And so now we have substitutions for both of the factors in the argument of the sine function. So we plug in 2 Pie f in place of Omega and one over four f in place of the time and so the final magnetic field is going to be B naught times sine of Pie over two because the f cancels and two over four is one over two and then there's a Pie factor in the top there. And the initial magnetic field will be zero because we’ll take time initial time to be time zero in which case sine of zero is zero. So the induced EMF then is the number of turns times the area of the coil times the final magnetic field B naught times sine Pie over two and times the area of the coil Pie times diameter squared over four divided by the time which is one over four f. That's what we figured out right here. And so multiply top and bottom by four f to make this look a little bit cleaner instead of having fractions within fractions and we have number of turns times Pie times diameter squared times frequency times Peak magnetic field strengths times sine of Pie over two is the EMF induced in a quarter cycle. So it’s 200 times Pie times 30 times ten to the minus two meters squared times 100 times ten to the six Hertz times one times ten to the negative 12 Tesla times one because that's what sine of Pie over two is and that makes a five point six five times ten to the minus three volts. Then part c asks us for the capacitance that this circuit must have given a certain inductance of 2.5 micro henry’s and the frequency of 100 megahertz. So the resonant frequency is one over two Pie times the square root of the inductance times the capacitance and then we can square both sides to get the next line, which is resonant frequency squared equals one over four Pie squared LC and then we'll solve for C by multiplying both sides by C over resonant frequency squared. And we get C is one over four Pie squared L f naught squared. So it's one over four Pie squared times 2.5 times ten to the minus six Henry's times 100 times ten to the six Hertz squared which gives a capacitance of 1.01 times ten to the minus 12 farads.