Question
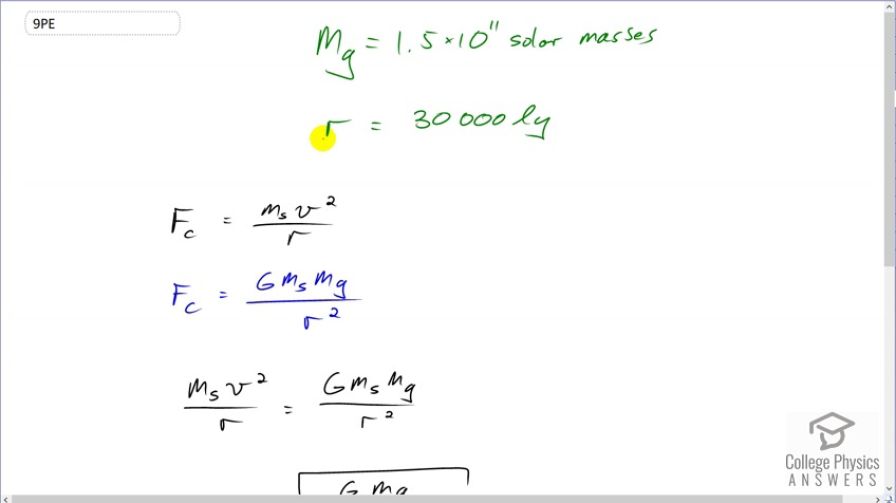
Assuming a circular orbit for the Sun about the center of the Milky Way galaxy, calculate its orbital speed using the following information: The mass of the galaxy is equivalent to a single mass times that of the Sun (or ), located 30,000 ly away.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 34, Problem 9 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We assume the Sun is orbiting a point mass of mass 1.5 times 10 to the 11 solar masses at a distance of 30,000 light years. So we are taking an average of all the milky way galaxy's stars positions and masses and averaging it out to being a point mass at this distance of this mass. So the centripetal force of the Sun experiences is the Sun's mass times its speed squared divided by its distance from the center and that force is being provided by gravity so you can also express it in terms of the gravitation equation which is the gravitational constant times the mass of the Sun times the mass of the galaxy divided by the distance from the center squared. So these are both the centripetal force and so they can be equated and the Sun's mass cancels and multiply both sides by r and so this is now r to the power of 1 in the denominator and then take the square root of both sides and you get that the speed of the Sun then in orbit around the center of the galaxy is square root of the gravitational constant times the mass of the galaxy divided by the galaxy's radius. So that's the square root of gravitational constant times the mass of the galaxy which is 1.5 times 10 to the 11 solar masses and we convert that into kilograms by multiplying by the mass of the Sun. divided by the distance which we have to express in in meters so that we can have meters per second as our final answer. So it's 30,000 light years times the speed of light times a year in seconds. There. So 2.6 times 10 to the 5 meters per second is the speed at which the Sun is orbiting the center of the galaxy.
