Question
Repeat Exercise 5.14 for a car with four-wheel drive.
Exercise 5.14
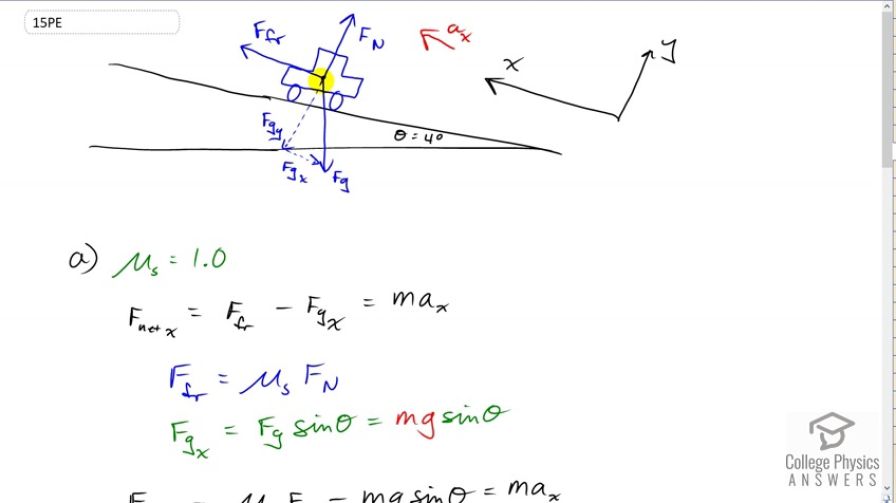
Calculate the maximum acceleration of a car that is heading up a slope (one that makes an angle of with the horizontal) under the following road conditions. Assume that only half the weight of the car is supported by the two drive wheels and that the coefficient of static friction is involved—that is, the tires are not allowed to slip during the acceleration. (Ignore rolling.) (a) On dry concrete. (b) On wet concrete. (c) On ice, assuming that , the same as for shoes on ice.Final Answer
- It will slide down the hill!
Solution video
OpenStax College Physics for AP® Courses, Chapter 5, Problem 15 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
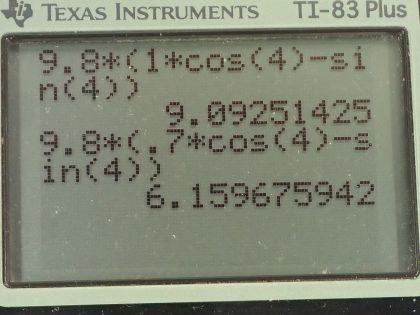
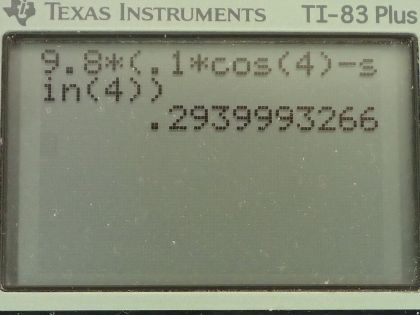
This is College Physics Answers with Shaun Dychko. This car on a slope is accelerating up the slope and there will be a force of friction applied upwards on the slope and there is going to be a component of gravity pulling it back down the slope and there is a normal force and a component of gravity perpendicular to the slope. I’ve rotated the coordinate system and also flipped it around so that x is positive up the slope and y is positive perpendicular and away from the slope. The slope has an incline of four degrees and we have to consider different conditions. The first being rubber on dry concrete, the second is rubber on wet concrete for a coefficient of static friction of 0.7, and lastly rubber on icy concrete which is going to have a coefficient of static friction of 0.1. So what will the acceleration of the car be in each case. On the x direction, we know that the friction force is positive because it’s going up the slope and we have to find x to be positive in that direction, minus the component of gravity in the x direction and it’s directed down the slope. That equals mass times acceleration in the x direction. The force of friction will be the coefficient of static friction multiplied by the normal force and the Fgx is going to be Fg times sin theta because this angle in here is equal to theta. We know that because if you consider this triangle here, it’s a right triangle and this angle plus theta has to add up to 90 since this angle down here is 90 and all the angles in the triangle add up to 180. That leaves 90 leftover for these two and this angle which I’ve labelled with a dot, plus theta has to equal 90, and this dot angle plus this angle here also has to make a 90 since Fgy is also perpendicular to the slope. So that means this must be theta if dot plus this thing has to be 90 and dot plus that thing has to be 90, then this equals that. So we have the x component of gravity then is Fg times sine of theta because this Fgx is the opposite leg of this blue triangle. I’ve substituted mg in place of Fg there too. So we replaced some terms in our Fnet x formula, force of friction is mu s times Fn and force of gravity in the x direction is mg sin theta. That equals mass times acceleration in the x direction. Now we need to also substitute for the normal force, so we consider the y direction now. We know that the net force in the y direction is zero because there is no acceleration perpendicular to the slope. So that means Fn which is positive y minus Fgy equals zero. That means Fn equals Fgy and Fgy is mg times cos theta because Fgy is the adjacent leg of this blue right triangle. So we substitute mg cos theta in place of Fn here. So we have mu s mg cos theta minus mg sin theta equals ma x and we can divide both sides by m and the m cancels on both sides, and we have a x equals g which I’ve factored out between these two terms, times bracket coefficient of static friction times cos theta minus sin theta. So we have 9.8 meters per second squared for g, times 1.0 the coefficient of static friction between rubber and dry concrete times cos 4 minus sin 4 is 9.1 meters per second squared will be the maximum possible acceleration up the slope. Then in part b, given a coefficient of static friction of 0.7 between rubber and wet concrete, we end up with a maximum acceleration of 6.2 meters per second squared. And then for icy slope, we have a coefficient of static friction of 0.1, and this gives 0.3 meters per second squared acceleration up the slope will be the maximum.