Question
A farmer making grape juice fills a glass bottle to the brim and caps it tightly. The juice expands more than the glass when it warms up, in such a way that the volume increases by 0.2% (that is, ) relative to the space available. Calculate the magnitude of the normal force exerted by the juice per square centimeter if its bulk modulus is , assuming the bottle does not break. In view of your answer, do you think the bottle will survive?
Final Answer
. This is like supported by of glass. It will probably break.
Solution video
OpenStax College Physics for AP® Courses, Chapter 5, Problem 41 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
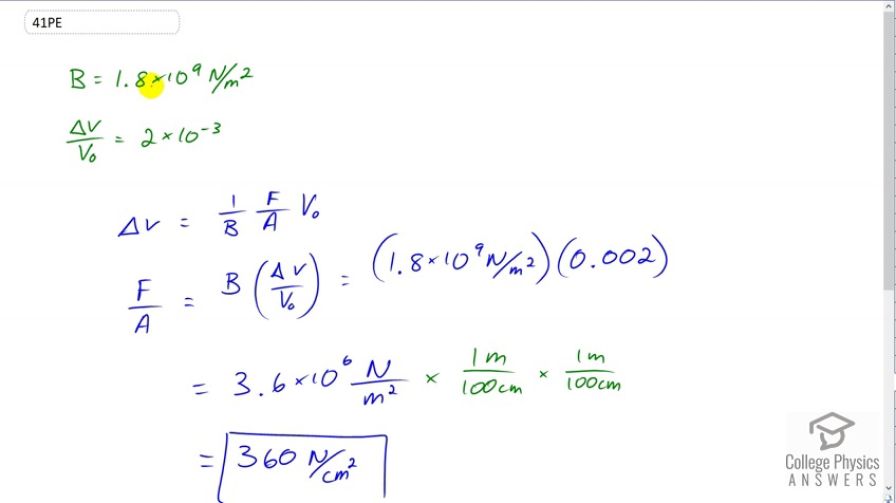
This is College Physics Answers with Shaun Dychko. We begin this question by writing down the information that we're given. We're told that the bulk modulus of the glass used to make this grape juice bottle is 1.8 times ten to the nine newtons per square meter and that the fractional change of volume is two times ten to the minus three. So we have this formula that tells us that the change in volume of the container equals one over the bulk modulus, times the force per area on the surface of the object, multiplied by its original volume. We can divide both sides by v naught and multiply both sides by B and that isolates f over a which is what we want to find. Then we'll switch the sides of the equation around as well. We have force per area equals bulk modulus times the change in volume divided by the original volume. So that's 1.8 times ten to the nine newtons per square meter, times 0.002 which is 0.2 percent written as a decimal. This gives 3.6 times ten to the six newtons per square meter. This shows why it's really useful to write units in your work because then you notice at the end, we have these units and this is not what the question is asking for. It is asking for newtons per square centimeter instead. So we have to multiply by one meter for every 100 centimeters, which takes care of one of the two meters that are being multiplied together here. Then multiply again by one meter for every 100 centimeters which fully cancels the meter squared there. Now we have centimeters squared in the denominator and 3.6 times ten to the six divided by 100, times 100 is 360 newtons per square centimeter. Now, 360 newtons if you divide by gravitational field strength, 9.8 newtons per kilogram is similar to supporting a mass of 36 kilograms using one square centimeter of glass. I converted it into kilograms because we have sense of how heavy something with a mass of 36 kilograms would seem or feel like. It's really heavy and supporting it with one square centimeter of glass does not seem realistic. So the glass would probably break.