Question
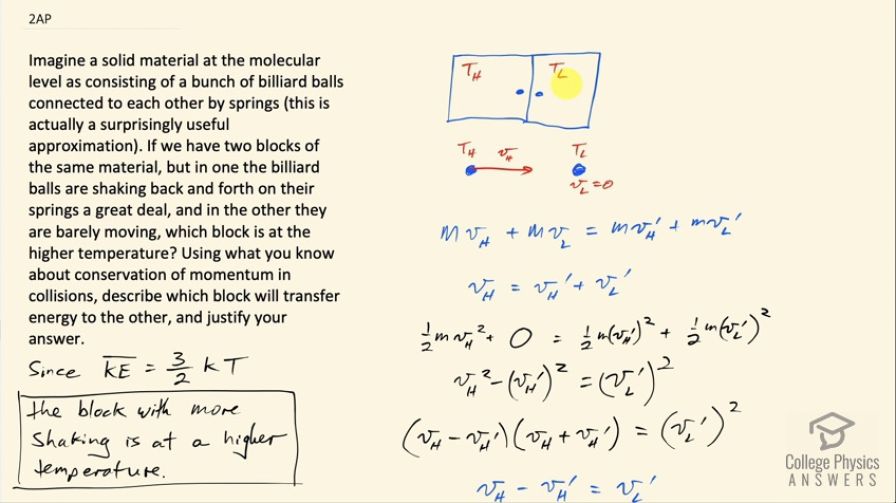
Imagine a solid material at the molecular level as consisting of a bunch of billiard balls connected to each other by springs (this is actually a surprisingly useful approximation). If we have two blocks of the same material, but in one the billiard balls are shaking back and forth on their springs a great deal, and in the other they are barely moving, which block is at the higher temperature? Using what you know about conservation of momentum in collisions, describe which block will transfer energy to the other, and justify your answer.
Final Answer
The block with more shaking is at a higher temperature. The energy transfer will be greater from the high temperature balls to the low temperature balls. Please see the solution video for explanations.
Solution video
OpenStax College Physics for AP® Courses, Chapter 15, Problem 2 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. We have two blocks of the same material and one block has a high temperature and the other block has a low temperature and we know that because we are told that the block or the billiard balls that we use to imagine the molecules in the high temperature block are moving faster... we are told that their moving back and forth on their imaginary spring is a great deal and the other block not as much. So we know from chapter 13 that the average kinetic energy, which is related to their speed because kinetic energy is one-half mv squared is 3 over 2 times Boltzmann's constant times the temperature. So the temperature is related to the kinetic energy so the block with more shaking is at higher temperature. Okay! Then we are going to talk about conservation of momentum and collisions between billiard balls so to speak between these two materials and talk about how energy will be transferred. So if we have this collision here, we'll imagine that the low temperature billiard ball is such low temperature that it has a speed of zero initially before the collision and we'll say that just to make our algebra a little bit easier and we'll have this high temperature billiard ball coming in for collision this collision would be elastic as we would expect you know, if there's springs in between these balls, the springs would absorb some of that kinetic energy and then release it and so the total kinetic energy would remain the same after the collision. Okay! So this is saying the total momentum before the collision equals the total momentum after the collision the momentum of the low temperature ball is zero initially and so we have this here after we divide both sides by m. Since the material is the same in the two blocks that means the mass of every molecule or every billiard ball is the same so there's no need for a subscript H or L in the m factor and so we can cancel it out everywhere and we are left with v H equals v H prime plus v L prime so these are the speeds of the high-temperature billiard ball and low temperature billiard ball respectively after collision. Since the collision is elastic, the one-half mass times the high speed ball initially squared plus zero since the low temperature billiard ball has no kinetic energy initially it's gonna equal the total kinetic energy of the high temperature and low temperature balls after collision and we can rearrange this after multiplying both sides by 2 over m, we can rearrange this to what we have: v H squared minus v H prime squared equals v L prime squared so after doing this multiplying and then I subtracted v H prime squared from both sides. Okay! And doing it this way allows us to factor the left side because there's a common pattern when you have a difference of two things squared you can write this difference of squares as the product of two binomials— one where you have the terms added and the other binomial where you have the term subtracted— so it's v H minus v H prime times v H plus v H prime is the same as v H squared minus v H prime squared and all of this equals v L prime squared and the reason we did that is because we can rearrange this formula from conservation of momentum and write it as v H minus v H prime equals v L by subtracting v H prime from both sides and then we can substitute for this with v L prime because v L prime is v H minus v H prime so we write v L prime there instead times v H plus v H prime equals v L prime squared and then divide both sides by v L prime and then we get v L prime is v H plus v H prime and this can in turn be substituted back into the conservation of momentum formula for v L prime and write v H plus v H prime there instead and then we combine the two v H prime's to get 2v H prime and then minus v H from both sides giving us zero on the other side and so 2v H prime equals zero in which case v H prime is zero and this tells us that kinetic energy is getting transferred from the incoming ball, which then becomes at rest after the collision to the other ball and this energy transfer will be greater from the high temperature balls to the low temperature balls because the high temperature balls are moving with a higher initial kinetic energy and... there we go!