Question
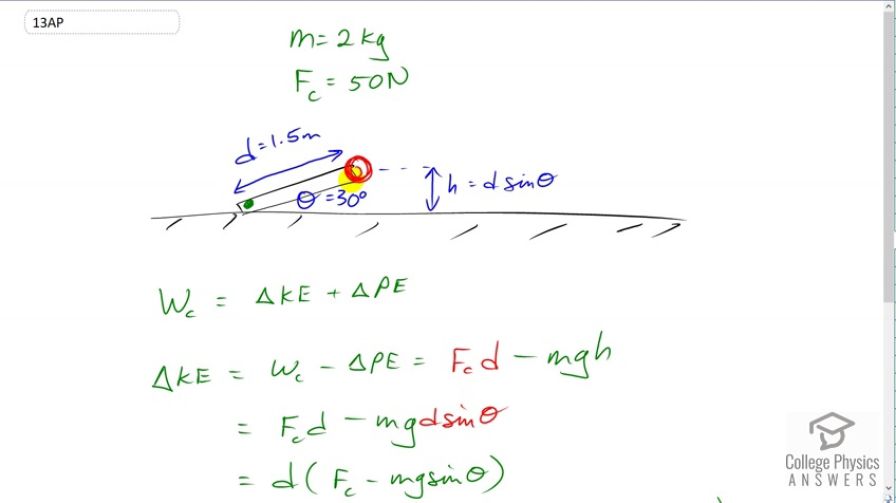
You are launching a 2-kg potato out of a potato cannon. The cannon is 1.5 m long and is aimed 30 degrees above the horizontal. It exerts a 50 N force on the potato. What is the kinetic energy of the potato as it leaves the muzzle of the potato cannon?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 7, Problem 13 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
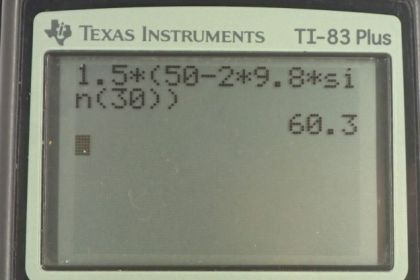
This is College Physics Answers with Shaun Dychko. The mechanical energy gained by the potato by this point at the muzzle of the potato cannon has to be provided by the work done by the cannon. The work done by the cannon is the force applied by the cannon, which is 50 newtons multiplied by the length of the cannon which I've labeled d. This work done by the cannon will turn into kinetic energy of the potato as well as potential energy of the potato. This question asks us to find out what is the change in kinetic energy. Well, it says what is the final kinetic energy that it has at the muzzle but since it starts with zero kinetic energy, this change in kinetic energy is the final kinetic energy. So delta kE equals the work done by the cannon minus the energy gained through gravitational potential energy. So that's Fc d work done by the cannon minus m g h, change in potential energy, and h is this height here which is the opposite leg of this yellow triangle here. We're given this angle theta of 30 degrees and so we can find h by going the hypotenuse d multiplied by sine theta. So we substitute that for h and then factor out the common factor d and we have our kinetic energy is going to be the length of the potato cannon, times by the force applied by the cannon, minus the weight of the potato, times sine theta. So it's 1.5 meters, times 50 newtons, minus two kilograms, times 9.8 newtons per kilogram, times sine 30, which is 60 Joules.