Question
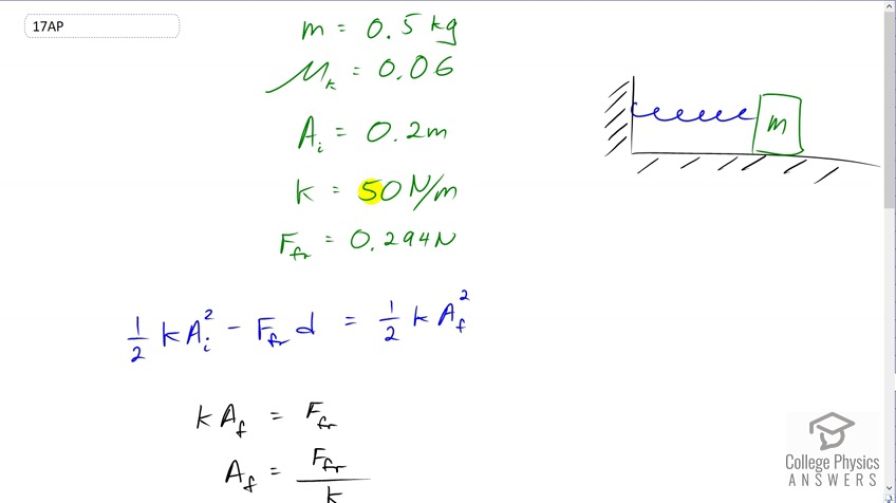
A 0.5-kg object is connected to a spring that undergoes oscillatory motion. There is friction between the object and the surface it is kept on given by coefficient of friction . If the object is released 0.2 m from equilibrium, what is the distance that the object travels? Given that the force constant of the spring is 50 N/m and the frictional force between the objects is 0.294 N.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 16, Problem 17 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We have an object connected to a spring that's going to be sliding on horizontal surface. And there's a coefficient of friction of 0.06, that's kinetic friction because it is moving and sliding. And the object has a mass of 0.5 kilograms and is released from a position that is 0.2 meters from the equilibrium position which means that its amplitude of its oscillation begins at 0.2 meters. And I call this A subscript i for initial. And the spring constant is 50 newtons per meter. And the question is just helpful in telling us what the friction force is but we could have calculated it. It would have been mu mg but mu k times m times g works out to 0.294 newtons. So, we know that initially there is some elastic potential energy equal to one half times the spring constant times the initial amplitude squared. And that's the total energy that the system has to begin with. And then over time some of that energy will be dissipated by friction and in an amount of the friction force multiplied by the total distance over which the mass is sliding. And then when you take this energy dissipated by friction away from the total initial energy, you left with the total elastic potential energy that the system has when the mass has finally comes to rest and at a position it has in a final amplitude called A subscript f. So, we need to figure out what Af is because everything else we know here except for d. d is what we're trying to solve for, the total distance that the mass slides over. And when the mass finally comes to rest, it will do so when the maximum displacement from equilibrium is A subscript f. And when that multiplied by spring constant equals the friction force because at this point it won't be able to slide anymore because the spring, in order to make the mass move, the spring force needs to exceed friction but at this point it will stop moving because it equals friction. And we solve for Af by dividing both sides by k. And so the final amplitude when the mass stops is the friction force divided by k. So we substitute that in for Af. And now, our job is to solve for d. So we'll add force friction times d to both sides. And then we'll also subtract the one half times friction force squared over k from both sides. And I write friction force over k because we have friction force squared over k squared multiplied by k and this works out to k to the power of one in the denominator. And then we switch the sides around to get the unknown on the left and we have force of friction times d is one half which we factored out from both of these times k times initial amplitude squared minus friction force squared over k. Then we divide both sides by the friction force and we get the total distance equals one over two, force of friction times kAi squared minus force friction squared over k. And then we substitute in numbers. So we have one over two times 0.294 newtons times 50 newtons per meters spring constant times an initial amplitude of 0.2 meters squared minus 0.294 newtons squared over 50 newtons per meter. And this works out to 3.40 meters as a total distance the mass would slide before coming to rest.
