Question
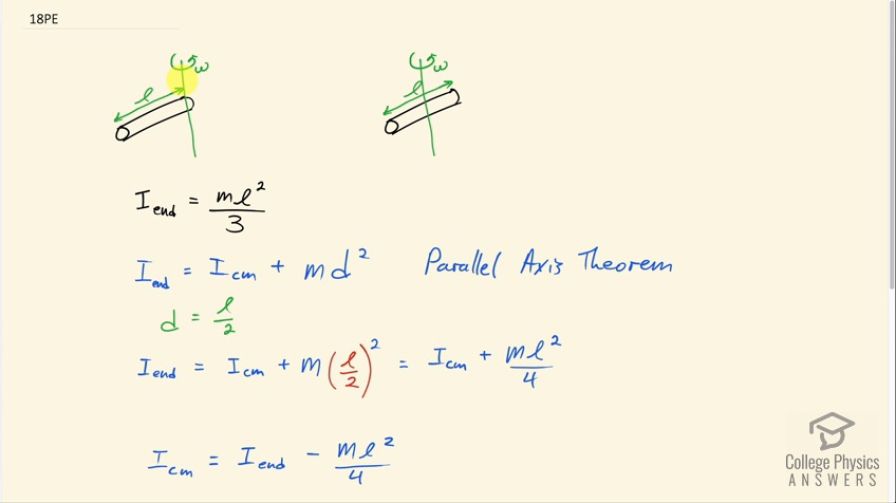
Starting with the formula for the moment of inertia of a rod rotated around an axis through one end perpendicular to its length , prove that the moment of inertia of a rod rotated about an axis through its center perpendicular to its length is . You will find the graphics in Figure 10.12 useful in visualizing these rotations.
You will need the parallel axis theorem, a derivation of which is offered by AK Lectures.
Final Answer
Please see the solution video.
Solution video
OpenStax College Physics for AP® Courses, Chapter 10, Problem 18 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. This question says given this formula for the moment of inertia of a thin rod with an axis of rotation at an end perpendicular to the length so that's this picture here where the rod is gonna go around this green line. Given this information we know, figure out what the moment of inertia would be in this case where the line goes through the center of the rod; this would be going through the center of mass. So there's something called the parallel axis theorem that I don't think the textbook really even mentions but it's the way to solve this question and I have put a link to this other guy aka who derives this and it says that the moment of inertia about any axis such as 'on the end' or any axis at all along this rod so long as the axis is parallel to this one here, it's going to equal the moment of inertia at the center of mass plus the mass of this whole rod multiplied by the distance between the center of mass axis and the axis that we are interested in such as at the end. So it's a way to find the moment of inertia given that you know the moment of inertia about the center of mass and you know the mass of the thing and this distance between the two parallel axis of rotation. Now this is what we wanna find but we know this one because we are told we can assume that ml squared over 3 is the moment of inertia of the end and so we can rearrange this to solve for the center of mass moment of inertia. Now this distance between the axis is going to be half the length because this center of mass is in the geometric center of the rod and the distance to the end is l over 2 so d gets replaced with l over 2 here. And so moment of inertia at the end is moment of inertia of the center of mass plus ml squared over 4. Then we subtract ml squared over 4 from both sides and then switch the sides around and we get the center of mass moment of inertia is the moment of inertia with an axis at the end minus ml squared over 4. And then this can be replaced with what we know— ml squared over 3— and then we, you know, subtract these fractions. So multiply top and bottom by 4, multiply top and bottom by 3 and we get 4ml squared over 12 minus 3ml squared over 12 is ml squared over 12. So this is the moment of inertia for an axis through the center of mass that is perpendicular to the length of the rod.