Question
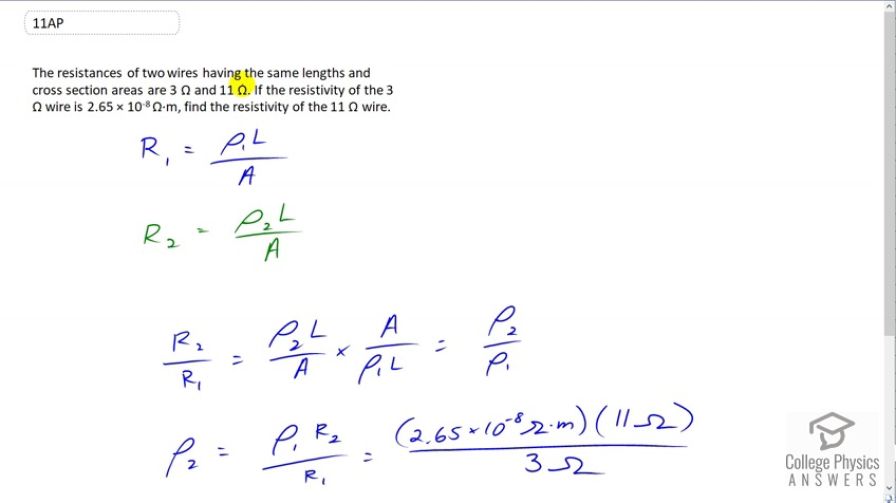
The resistances of two wires having the same lengths and cross section areas are 3 Ω and 11 Ω. If the resistivity of the 3 Ω wire is 2.65 × 10−8 Ω·m, find the resistivity of the 11 Ω wire.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 20, Problem 11 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We have two wires, each with the same length and the same cross-sectional area and they have different resistances, three ohms and 11 ohms. We're given the resistivity of one of the wires and we have to find the resistivity of the other. So resistance of wire one will be the resistivity of the material that wire one is made of, multiplied by its length divided by its area. I did not put a subscript one on the length or area factors because we're told that those things are the same between the two wires. So resistance for wire two is going to be resistivity number two multiplied by length -- the same length and divided by the same area and so when we divide these resistances, we take resistance two and instead of dividing a fraction by a fraction I'm multiplying by the reciprocal of the denominator here, so multiplying by A over rho one l. The A's and l's cancel leaving us with rho two over rho one. So we solve for resistivity two by multiplying both sides by resistivity one and we get resistivity two is resistivity one times resistance two divided by resistance one. That is 2.65 times ten to the minus eight ohm meters, resistivity for material one, multiplied by 11 ohms resistance of the second wire divided by three ohm resistance of the first wire. This gives 9.7 times ten to the minus eight ohm meters for the resistivity of the second resistor.
