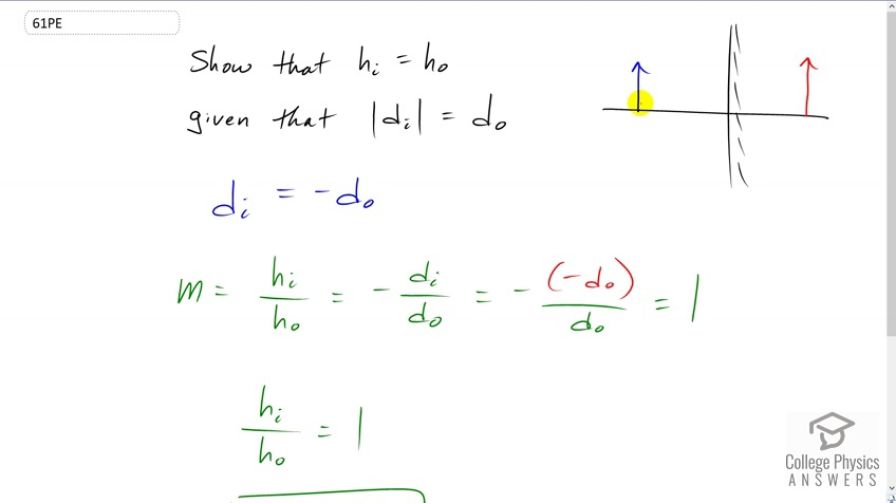
Question
Show that for a flat mirror , knowing that the
image is a distance behind the mirror equal in magnitude to the distance of the object from the mirror.
Final Answer
Please watch the solution video.
Solution video
OpenStax College Physics for AP® Courses, Chapter 25, Problem 61 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. We're going to show that the image height is the same as the object height given a flat mirror. And, knowing that the object distance is of equal size to the image distance. Now, given that these are equal sizes and knowing that this image is on the other side of the mirror compared to the object, this must be a virtual image because the light rays do not pass through this image. Instead, they're blocked by the mirror then reflected. And so, we can say that this image distance is going to be the negative of the object distance. So, we take the object distance to be positive and the image distance will have the opposite sign and it will be negative since it's a virtual image. And so, this is what we can substitute into our magnification formula and we have magnification is image height divided by object height and that's the negative of image distance over object distance, and then we substitute negative D object in place of D image. And, this negative and a negative makes positive one, and the Dos cancel, and we have image height over object height is one, in which case after you multiply both sides by object height, you find that the image height equals the object height.