Question
A car engine moves a piston with a circular cross section of diameter a distance of to compress the gas in the cylinder. (a) By what amount is the gas decreased in volume in cubic centimeters? (b) Find the uncertainty in this volume.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 1, Problem 28 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
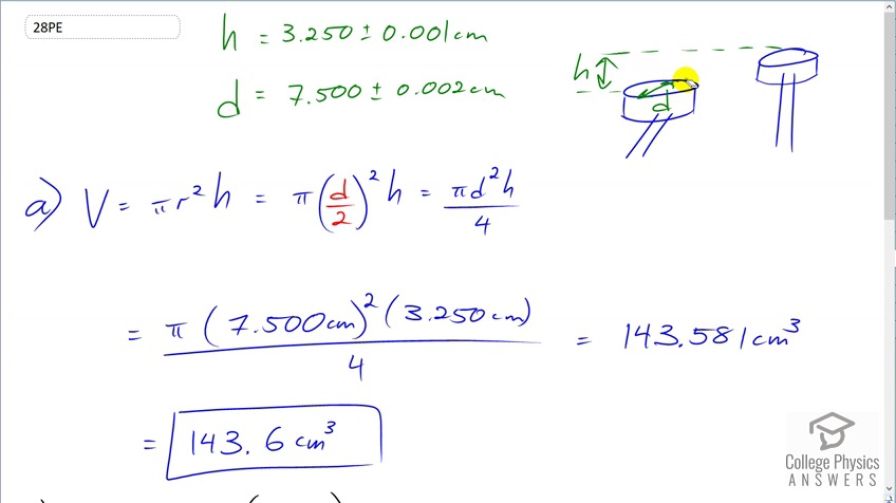
This is College Physics Answers with Shaun Dychko. The piston in a car engine is compressing some air-gas mixture here and the piston has a cross-sectional diameter of 7.500 plus or minus 0.002 centimeters and it's going to be going up this height of 3.250 plus or minus 0.001 centimeters and the question is what change in volume is there in this gas? Well, we have to find the volume of this cylinder of space that's above the piston and so that's going to be the area of one of the ends and multiplied by the height. So the area of one of the ends is π times radius squared and we are not given the radius, we are given the diameter so we substitute d over 2 in place of r and then square that results in the formula π times d squared times h divided by 4. And so we are gonna plug in numbers and get an answer but we are not going to really know what we should round this answer to until we do part (b) which gives us the uncertainty in the volume. Okay. So we have π times the diameter of 7.500 centimeters squared times the height it goes up, 3.250 centimeters, divide by 4 we get 143.581 cubic centimeters and using our, sort of, back-of-the-envelope rule where we figure out which number in this product has the least number of significant figures and they all have four so this height and this diameter each have four centimeters so we are gonna choose to have four significant figures, I should say, in our answer, 143.6. Now that's sort of a rough guess of how many significant figures we should have and usually that is the technique you will use but in this particular question, we are given absolute errors and so there's a more sophisticated technique for determining how many significant figures we should have in our answer and we are gonna do that now in part (b). So first we figure out the percent uncertainty in the volume and when you are multiplying and dividing the percent uncertainty in your answer is going to be the percent uncertainty of the numbers you are working with each of the measurement's all added together. And so we have the percent uncertainty in this diameter plus the percent uncertainty in the diameter because we are multiplying it by itself and so that's why there is this multiply by 2 here and then add to that the percent uncertainty in this height so that's what this is saying here. So take the percent uncertainty in diameter and add it to itself once more which is the same as multiplying by 2 and then add also the percent uncertainty in the height and this will give us the percent uncertainty in the volume. So percent uncertainty in diameter is 0.002 centimeters— absolute uncertainty, that's this number here the plus or minus part— divided by the measurement of 7.500 times by a 100 percent times 2 plus the absolute uncertainty in the height divided by the height measurement and this gives 0.08410 percent uncertainty in the volume. We are not gonna round this to 0.08 percent although we would if that was meant to be our final answer but this is just an intermediate calculation and so we keep it unrounded and use it for this next calculation which is the absolute error in the volume. So that's going to be the volume calculation we did in part (a) multiplied by its percent uncertainty divided by 100 percent so that's 143.581 cubic centimeters times 0.08410 percent divided by 100 percent and that gives 0.121 cubic centimeters and we'll round that to one significant figure as we normally do for errors; sometimes you can keep two significant figures but in this case, we'll just keep one, you know, these errors here are given with one significant figure. So this means our volume can be stated to the tenths place and so that means we have to round this to 143.6 and that's what we did anyway... this back-of-the-envelope sort of technique where you just count the number of significant figures in your factors and then give the smallest number to the answer that usually works out the same as this more sophisticated analysis would say and there we go!

