Question
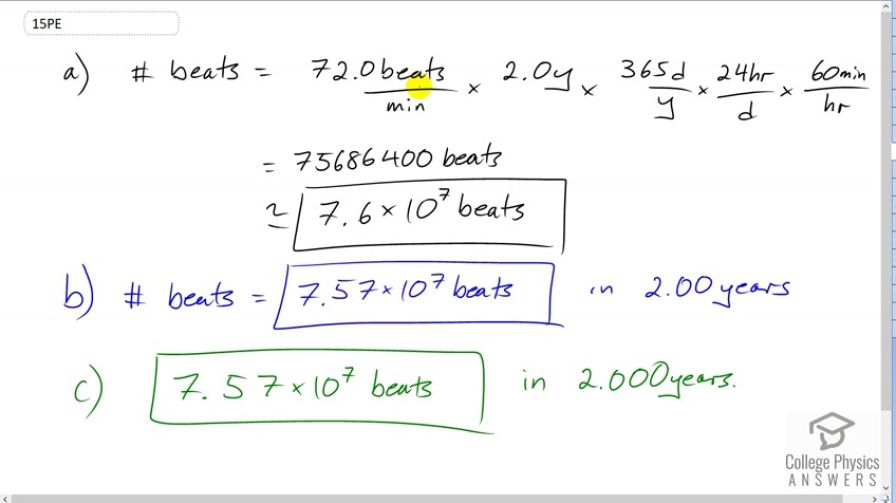
(a) Suppose that a person has an average heart rate of 72.0 beats/min. How many beats does he or she have in 2.0 y? (b) In 2.00 y? (c) In 2.000 y?
Final Answer
a)
b)
c)
Solution video
OpenStax College Physics, Chapter 1, Problem 15 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko We're going to calculate the number of heartbeats a person will experience in 2 years given the heart rate of 72.0 beats per minute. And we're going to do the calculation three times using the same number of years, which is 2, but the difference will be the amount of precision in our time period. So, we'll have 2.00 years in part B, and 2.000 years in part C. And, we'll see that our answer is the same quantity, but expressed with different precisions depending on the precision over time. Although in part C, they get a little bit tricky here actually. But, we'll get to that. So, first of all, in part A, we're going to multiply the 72.0 beats per minute by the time period of 2.0 years. Although, we're going to need some conversion factors in order to convert this years into a period of minutes, so that the minutes will cancel with the minutes in the denominator of our heart rate. So, we have 2.0 years times 365 days per year making the years cancel, leaving us with days at this point, for our time. But, that is still not good enough if we want minutes. So then, we'll multiply it by 24 hours per day and then multiply by 60 minutes per hour, leaving us finally with minutes in the top of our time period which cancel with the minutes in the denominator of our heart rate, leaving us with beats. So, that's 75,686,400 beats, but we're not going to express it to that precision because our time period has two significant digits and our heart rate has 72.0, that has three significant digits. And so, we... our answer, when you're multiplying, will have as many significant figures as the number with the least significant figures in your calculation, and that would the 2 years having only two significant figures. And so, we have only two significant figures in our answer here for part A. So, it's 7.6 times 10 to the 7 beats. For part B, we now are going to multiply it by 2.00 years. And so, the calculation is the same, but we're just putting 2.00 instead of 2.0. And so, we can have three significant figures now. Because we have three significant figures in our beats per minute, three significant figures in our time in part B. And so, we have 7.57 times 10 to the 7 beats. Now, notice I'm ignoring the fact that this is the number 24, which is a number with two significant figures. We can ignore that because this is a... this is an exact number. It's a conversion factor and there are precisely 24 hours in a day, basically by definition, and likewise for 60 minutes per hour. So, we don't need to think about the number of significant figures there. They are not measured numbers. These are, you could say, measured numbers. And so, it's the significant figures in these numbers that are important. Then in part C, we have four significant figures in our time period 2.000 years, and so, one might expect to have four significant figures in our final answer here, but no, because our heart rate has only three. And so, our precision is limited by the heart rate in part C. And so, we still repeat the same answer as part B: 7.57 times 10 to the 7 beats.
