Question
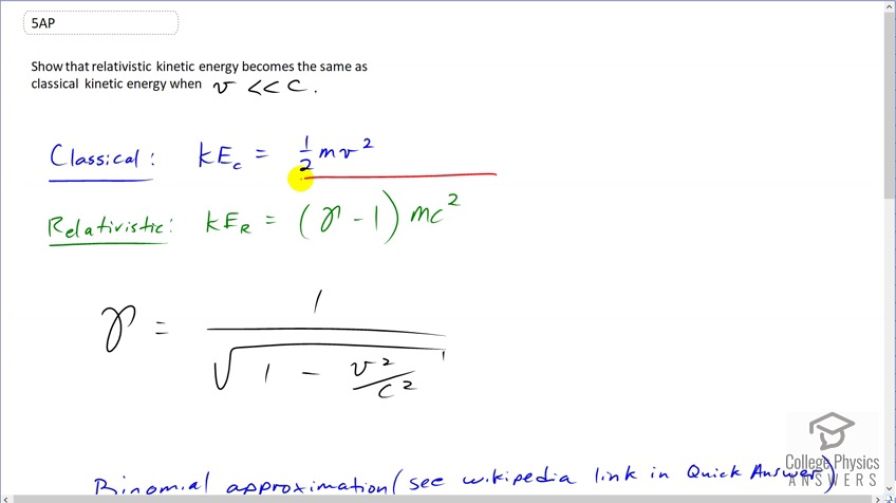
Show that relativistic kinetic energy becomes the same as classical kinetic energy when .
Final Answer
Please see the solution video, which uses the binomial approximation.
Solution video
OpenStax College Physics for AP® Courses, Chapter 28, Problem 5 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. We are going to show that when velocity is much much less than the speed of light, the relativistic kinetic energy formula, which is here, essentially becomes the same as the classical energy formula. So first let's make a substitution for gamma in this relativistic expression for kinetic energy; and so gamma is 1 divided by square root of 1 minus v squared over c squared. And when v is much much less than c, we can do the binomial approximation and you can look up how that works in the Wikipedia link in the quick answer above this video. And that says that, when you have a binomial, 1 plus some number x, if x is really small, and this is to some power alpha, when x is really small, we can say that this approximately equals 1 plus the exponent multiplied by this number x. So, in this case, we have gamma equals a binomial to the power of negative one-half and the negative is because I have to write this fraction as a thing to a single power and we can do that by raising this to the negative 1 which would make it square root 1 minus v squared over c squared to the power of negative 1. Now I have flipped the fraction and written it over 1 and it still doesn't quite look like this, we want a bracket and so we can do that by saying square root is another way of writing exponent one-half. So this is 1 minus v squared over c squared, to the power of one-half, all to the power of negative 1. And we can see we have a power to a power which means multiply the exponents together and so this is 1 minus v squared over c squared to the power of negative one-half. OK. So that's where this comes from. And our binomial approximation says, rewrite this as number 1 plus this exponent, whatever it is, negative one-half mutliplied by the number x, which is negative v squared over c squared because this binomial approximation says put a plus sign there so we can put a plus sign there by making this plus a negative. And so this negative and a negative makes a positive here and so we have 1 plus v squared over 2c squared. So gamma minus 1, if you subtract 1 from here and then 1 from here, is v squared over 2c squared and so gamma minus 1 is this and so we'll substitute v squared over 2c squared in its place, as I have shown here. And the c squared's cancel, giving us one-half m v squared, which is the classical kinetic energy formula. And this was possible because the binomial approximation works when x is really small or, in other words, when this v is much much less than c; when v is not much much less than c, the binomial approximation doesn't work anymore. There we go.