Question
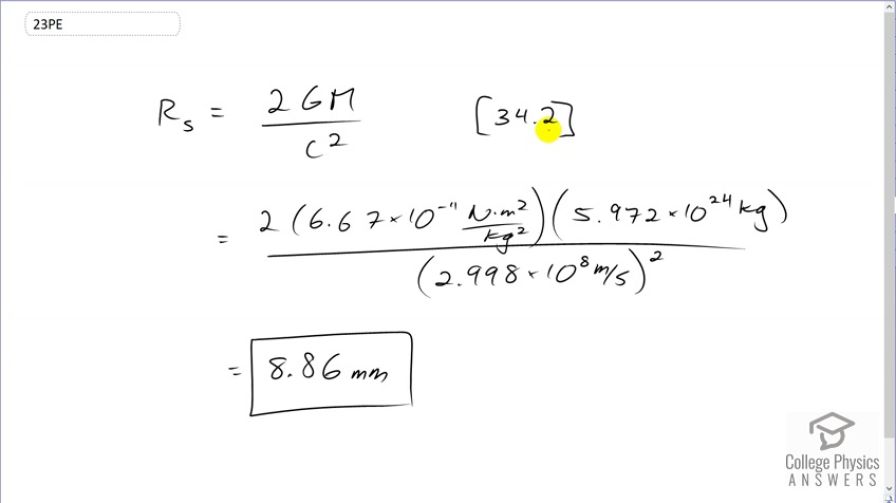
Black holes with masses smaller than those formed in supernovas may have been created in the Big Bang. Calculate the radius of one that has a mass equal to the Earth's.
Final Answer
No wonder they are so hard to observe!
Solution video
OpenStax College Physics, Chapter 34, Problem 23 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We are going to calculate the size of a black hole that has the mass of the Earth. So the Schwarzschild radius from equation 2 in chapter 34 it's 2 times the gravitational constant times the mass divided by c squared. So this is the distance to the event horizon around the black hole and so that's gonna be 2 times 6.67 times 10 to the minus 11 newton meter squared per kilogram squared times mass of the Earth divided by the speed of light squared giving a radius of 8.86 millimeters.
