Question
Suppose an MRI scanner uses 100-MHz radio waves. (a) Calculate the photon energy. (b) How does this compare to typical molecular binding energies?
Question by OpenStax is licensed under CC BY 4.0
Final Answer
  1. 4.14×10−7 eV4.14 \times 10^{-7}\textrm{ eV}
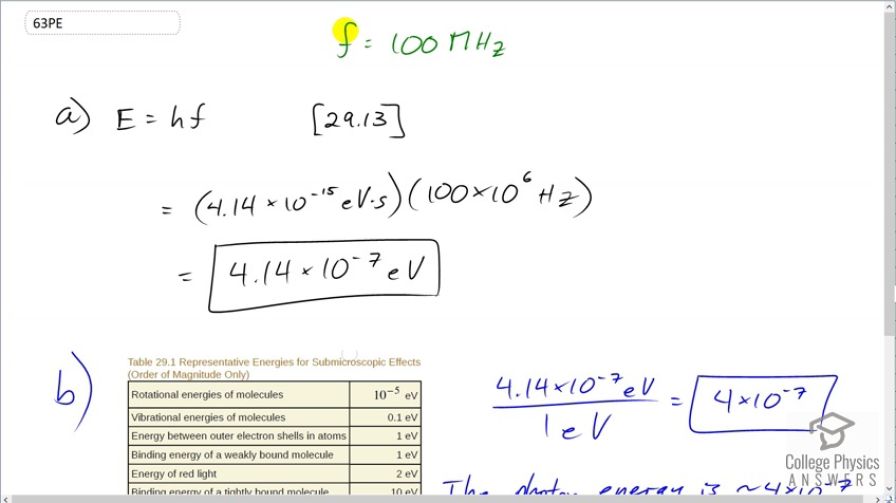
  2. The photon energy is about 4×10−74 \times 10^{-7} times smaller than typical molecular binding energies.

Solution video

OpenStax College Physics for AP® Courses, Chapter 30, Problem 63 (Problems & Exercises)

OpenStax College Physics, Chapter 30, Problem 63 (PE) video thumbnail

In order to watch this solution you need to have a subscription.

Start free trial Log in
vote with a rating of votes with an average rating of .
Chevron left Prev
Select problem
Next Chevron right

Calculator Screenshots

  • OpenStax College Physics, Chapter 30, Problem 63 (PE) calculator screenshot 1
Video Transcript
This is College Physics Answers with Shaun Dychko. The energy of a photon is given by equation [29.13] which is Planck's constant times the frequency and we are told the frequency is 100 megahertz. So we go 100 times 10 to the 6 hertz multiplied by Planck's constant— 4.14 times 10 to the minus 15 electron volt seconds— giving an energy of 4.14 times 10 to the minus 7 electron volts. And then we compare that with representative energies for sub-microscopic effects and we see that the binding energy of a weakly bound molecule is about 1 electron volt. And so we take this photon energy, divide it by that and we get 4 times 10 to the minus 7. So the photon energy is about 4 times 10 to the minus 7 times smaller than typical molecular binding energies.