Question
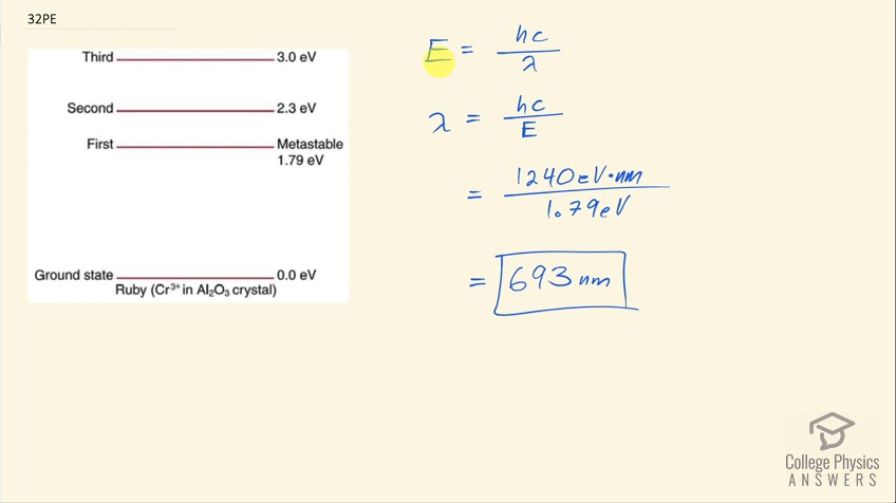
Ruby lasers have chromium atoms doped in an aluminum oxide crystal. The energy level diagram for chromium in a ruby is shown in Figure 30.64. What wavelength is emitted by a ruby laser?

Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 30, Problem 32 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. This question asks us what wavelength of light is emitted by a ruby laser? To answer that, we need to know what is the energy of the photons emitted and to figure that out we need to look at this energy level diagram for a ruby laser. We are told that this level here is metastable... in order to make a laser work, you need to have population inversion, which means you have a majority of electrons in the metastable state and much fewer in the ground state and then when electron of one atom makes a transition, it will emit a photon that then stimulates the electron in the atom beside it to also transition to the ground state and thereby emit its photon and so on and so on... there's this chain reaction and all of these in phase photons are constructively interfering and so on. Okay! So... it's the transition between the metastable state and the state below it that will result in the frequency emitted by the laser. So the energy then is 1.79 electron volts is going to be the energy of the photons emitted. So this energy is Planck's constant times speed of light divided by wavelength and we can solve for λ by multiplying both sides by λ divided by energy. And so we have then that λ is hc over E and Planck's constant times speed of light, we'll write as 1240 electron volt nanometers and divide that by the energy of the photons— 1.79 electron volts— and we'll get an answer in nanometers: 693 nanometers.
