Question
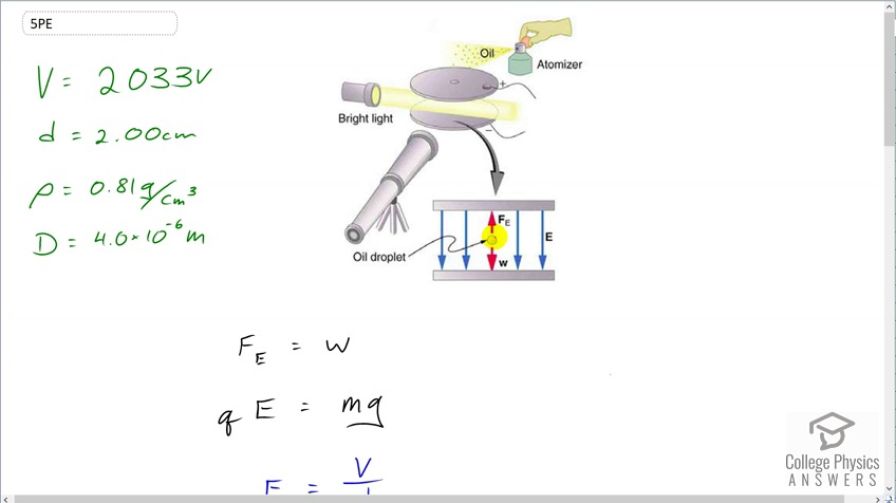
In Millikan’s oil-drop experiment, one looks at a small oil drop held motionless between two plates. Take the voltage between the plates to be 2033 V, and the plate separation to be 2.00 cm. The oil drop (of density ) has a diameter of . Find the charge on the drop, in terms of electron units.
Final Answer
16 charge units.
Note: at around 1:33 in the video I say "density", but I should be saying "diameter" of the droplet. The closed captions have the corrected text.
Solution video
OpenStax College Physics for AP® Courses, Chapter 30, Problem 5 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. This charged oil drop is suspended here between these parallel plates because of the electric field exerting a force upwards on it and the weight exerting an equal but oppositely-directed force downwards. So the electrostatic force equals the weight. And we can substitute for each these by saying the weight is mass times acceleration due to gravity. And then the electric field force is the charge on the oil drop times the strength of the electric field. Now the electric field we can figure out by going voltage divided by distance between the plates and we can substitute v over d then in place of E in this formula. And then we'll multiply both sides by d over V and then we solve for q, the charge. So it's gonna be the separation between plates times the mass of the droplet times gravitational field strength divided by the voltage. Now we are given a bunch of other data here; the diameter of the droplet, the density of the oil and well sorry, the diameter's this one here that's the diameter of the droplet with a capital D and so from this, we have to figure out the mass of the droplet. And so the density is defined as the mass per volume and the volume of a sphere is four-thirds π times radius cubed and we can write this as 3m over 4π r cubed multiplying both top and bottom by 3. And then we'll substitute diameter divided by 2 in place of radius because diameter is what we are given in this question and so we have 3m over 4π times diameter cubed and this is divided by 2 cubed which is 8. And then this 4 will cancel with that 8 leaving us with a 2 below the capital D cubed and then that 2 can be multiplied on top and bottom and we end up with 6m on top divided by π D cubed. So then we can solve for m here by multiplying both sides by π D cubed over 6. So m is π D cubed density divided by 6 and when we substitute that in place of m in our formula for the charge q. So we have q equals d times π times capital D—diameter—cubed times density times gravitational field strength divided by 6 times voltage. So a charge then is 2 times 10 to the minus 2 meters— separation between the plates— times π times 4 micrometers cubed times the density of oil converted into kilograms per cubic meter because we need mks units in our formulas to match with all other mks units that we have here that's meters, kilograms, seconds and then we multiply by 9.81 newtons per kilogram—gravitational field strength— divided by 6 times 2033 volts giving us this many coulombs. But the question says express your answer in number of charged units. And so we multiply this by 1 charge unit for every 1.602 times 10 to the minus 19 coulombs giving us 16 charge units.
