If a hydrogen atom has its electron in the $n=4$ state, how much energy in eV is needed to ionize it?

Video Transcript

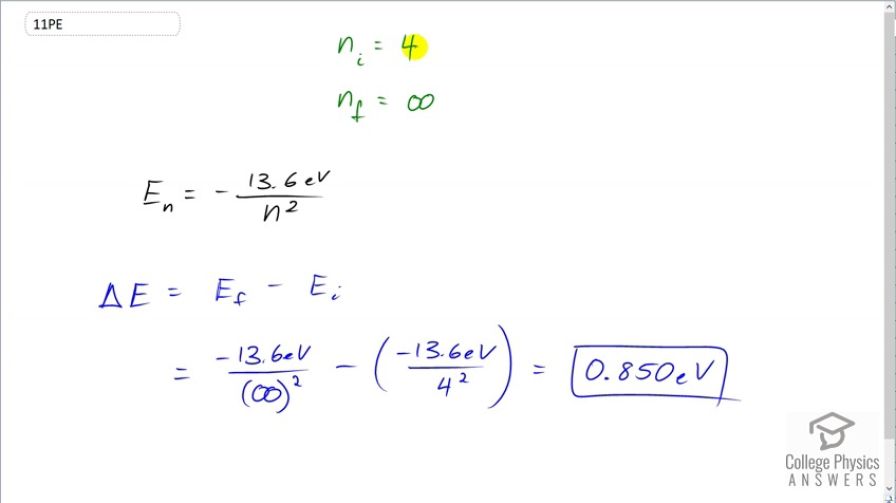
This is College Physics Answers with Shaun Dychko. This question asks how much energy is needed to ionize a hydrogen atom that has the electron in the n equals 4 state, initially. So that makes n i equal to 4 and the final state will have an value of n equal to infinity. That's what it means for an electron to be ionized, it's energy level is so big that it's no longer basically attached to the nucleus anymore. So the amount of energy of this final state will be negative 13.6 electron volts divided by infinity which essentially will make it zero. And so we have this difference in energy between the final state and the initial state is going to be zero minus the initial energy which is negative 13.6 electron volts divided by the initial energy level, 4 squared. This makes 0.850 electron volts. So that's the energy difference between the final state of the electron being energy level of infinity, or in other words, ionized versus electron being in a state, initially of 4 and this is the ionization energy then.