Question
Find the radius of a hydrogen atom in the state according to Bohr’s theory.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 30, Problem 13 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
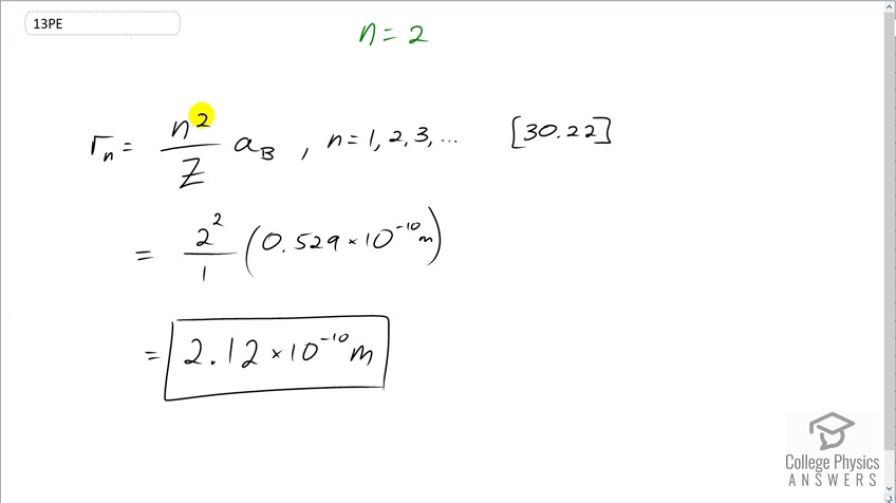
This is College Physics Answers with Shaun Dychko. According to Bohr's theory, the radius of a hydrogen-like atom is the principal quantum number squared divided by the atomic number or the number of protons in the nucleus, in other words, multiplied by the Bohr radius. And n can be any number from 1 up to infinity. So in this case, we are told n is 2 so we have 2 squared over 1; 1 because it's a hydrogen atom in which case, the atomic number is 1, 1 proton times 0.529 times 10 to the minus 10 meters— Bohr radius— which is 2.12 times 10 to the minus 10 meters will be the radius of this atom.
