Question
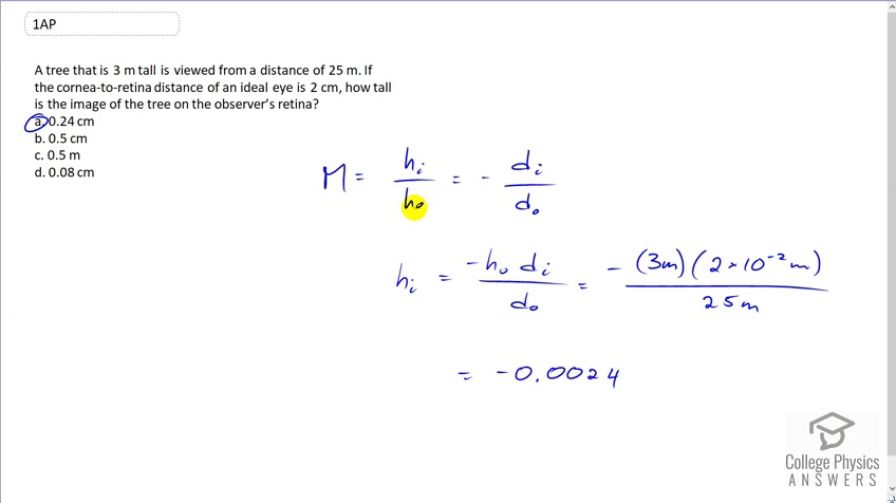
A tree that is 3 m tall is viewed from a distance of 25 m. If the cornea-to-retina distance of an ideal eye is 2 cm, how tall is the image of the tree on the observer’s retina?
- 0.24 cm
- 0.5 cm
- 0.5 m
- 0.08 cm
Final Answer
(a)
Solution video
OpenStax College Physics for AP® Courses, Chapter 26, Problem 1 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The height of the image on the person's retina is going to be divided by the height of the object in real life which is three meters and that ratio is the magnification. It also is the negative of the image distance divided by the object distance. Now, the image distance is the distance between the person's lens of their eye and their retina, which is two centimeters. And, the object distance that we're told is 25 meters. So, we can solve this for Hi by multiplying both sides by Ho. And so, the image height in the retina is going to be the negative of the object height multiplied by the image distance divided by the object distance. So, that's negative three meters times two centimeters written as times ten to the minus two meters divided by 25 meters, which is 0.0024 meters. And, this is 0.24 centimeters. So, the answer is A.
