Question
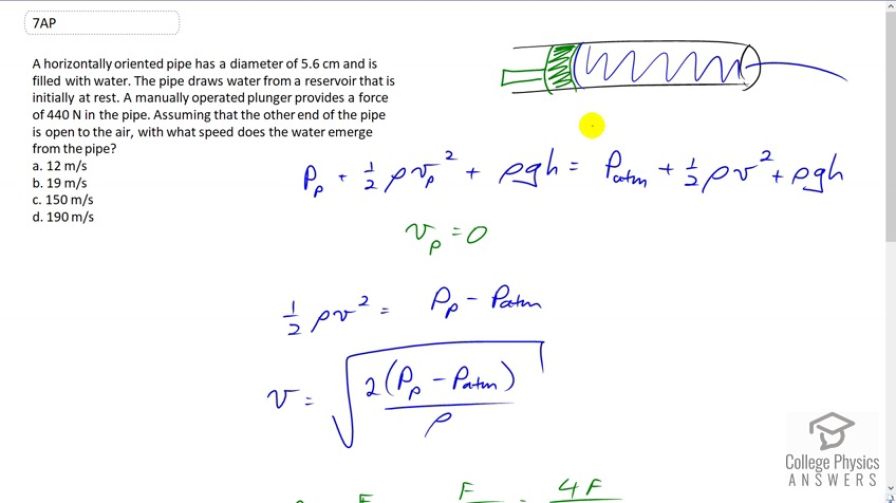
A horizontally oriented pipe has a diameter of 5.6 cm and is filled with water. The pipe draws water from a reservoir that is initially at rest. A manually operated plunger provides a force of 440 N in the pipe. Assuming that the other end of the pipe is open to the air, with what speed does the water emerge from the pipe?
- 12 m/s
- 19 m/s
- 150 m/s
- 190 m/s
Final Answer
(a)
Solution video
OpenStax College Physics for AP® Courses, Chapter 12, Problem 7 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We have to set up Bernoulli's Equation for this situation. We have a pipe with the diameter of 5.6 centimeters and is filled with water. And the pipe is taking water from the reservoir that's initially at rest. And then a plunger is put into one end of the pipe and it's squishing water at the other end. So for Bernoulli's Equation, we have this end where the plunger is, so we have subscript P here and we have this end which is the opening in the pipe. And our job is to figure out what is the speed with which the water is coming out of the pipe here. Now at the opening, the pressure is going to be atmospheric pressure because it's open to the air. And at the end where the plunger is, the pressure is going to be the pressure due to the plunger. And we're told that the plunger is being pressed with the force of 440 newtons. And that force is going to be spread over this cross-sectional area of the pipe. And so that's how we are going to find the pressure at the plunger. And we'll talk about that here. So, since the pipe is drawing water from the reservoir that is initially at rest that means this speed here of the water at the plunger is zero. And we also have this potential energy term which is the same for the both of them because it's the same for where it's open and it's the same where the plunger is because this pipe is horizontal. And so there's no difference in height. And so these terms are the same and so they cancel out. So we solve this for V after also making this term zero and we have one half rho V squared is the pressure at the plunger minus the atmospheric pressure of the opening. So subtracting Patm from both sides there. And then multiplied both sides by two over rho and then take the square root of both sides to solve for V. And then we'll make a substitution for the pressure at the plunger. It is the force supplied on the plunger divided by the cross-sectional area of the pipe and the area is Pi times diameter squared over four. That makes four F over Pi d squared and we substitute that in for P subscript P for plunger. And so the speed then at the opening is going to be the square root of two times four times the force divided by Pi times the tube's diameter squared minus the atmospheric pressure divided by the density of water. So we have square root two times four times four to the 40 newtons over Pi times 5.6 centimeters which is times ten to the minus two meters squared minus atmospheric pressure all divided by a thousand kilograms per cubic meter giving a speed of 12.4 meters per second. And so the answer is a.
